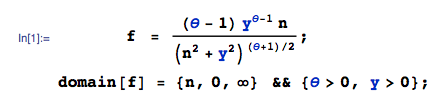In case you get stuck computing the integrals referred to in the above post, here is an automated way to proceed. Given random variable $N$ has pdf $f(n)$:
http://www.tri.org.au/se/mycustompdf.pngThe density is well-defined provided $\theta>1$. The mean $E_f[N]$ is:
http://www.tri.org.au/se/meancustompdf.pngand the variance of $N$ is:
http://www.tri.org.au/se/variancecustompdf.png where I am using the Expect and Var functions from the the mathStatica package for Mathematica to automate the nitty-gritties.
In the case of $\theta = 4$, the above results simplify to $E[N] = y$ and $Var(N) = y^2$.