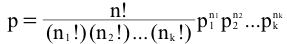You shouldn't use the binomial distribution here as it is a multinominal distribution problem (a generalization of the binomial).
So let's gather what we have:
n = 6 (total number of events)
n1 = 2 in part 1 (pudding #1)
n2 = 2 in part 2 (pudding #2)
n3 = 2 in part 3 (pudding #3)
p1 = 2/6 (probability to get 2 from n1)
p2 = 2/6 (probability to get 2 from n2)
p3 = 2/6 (probability to get 2 from n3)
the formula goes like this:
so let's put the numbers in motion:
$p = \frac{6!}{(2!*2!*2!)} *(2/6)^2 * (2/6)^2 * (2/6)^2 = 0.12345$$p = \frac{6!}{(2!*2!*2!)} *(\frac{2}{6})^2 * (\frac{2}{6})^2 * (\frac{2}{6})^2 = 0.12345 $
and we get
10/81