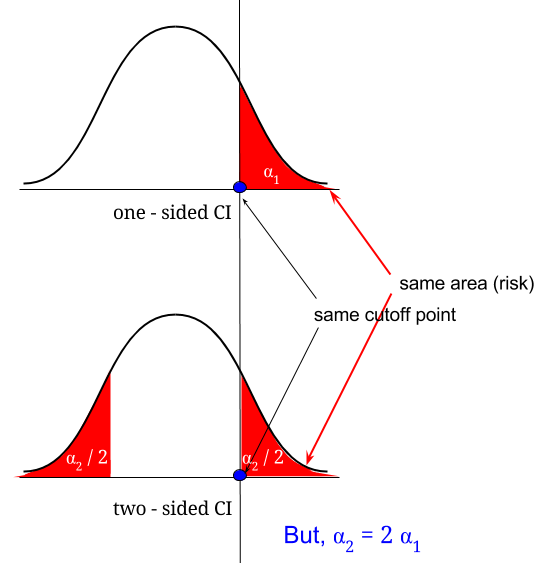
If you generate the two-sided confidence interval with a confidence level of $95\%$ (or $\alpha_1 = 5\%$), the cut-off points (or endpoints) of the interval will leave out a probability of a type I error of $\frac{1}{2} \alpha=2.5\%$ on either end.
If you are performing a one-sided test, and want to preserve a risk $\alpha = 5\%$ of rejecting the null when it is in fact true, you will want to generate a two-sided CI with and confidence level of $90\%$ to leave $5\%$ probability at each end.
So you double the initial $\alpha_1 =5\%$ to $\alpha_2=2\alpha_1=10\%.$
Hence the quote:
- When testing a two-sided hypothesis at the alpha level, use a $100(1 - \alpha )\%$ confidence interval.
- When testing a one-sided hypothesis at the alpha level, use a $100(1 - 2 \alpha)\%$ confidence interval.