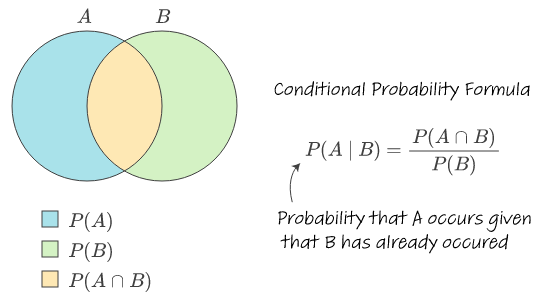
Quite a simple or at least short question: Why is $ \frac{P(A \cap B)}{P(B)} $ divided by $ P(B) $ for the conditional probability?

$ P(A | B) = \frac{P(A \cap B)}{P(B)} $

Random image to visualize:

Quite a simple or at least short question: Why is $ \frac{P(A \cap B)}{P(B)} $ divided by $ P(B) $ for the conditional probability?

$ P(A | B) = \frac{P(A \cap B)}{P(B)} $

Random image to visualize:

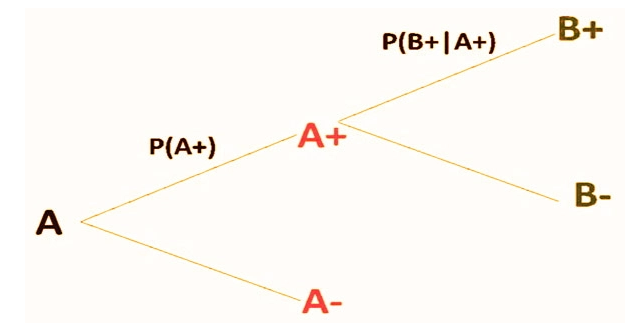
I actually would like to make use out of the tree display which I can't grasp at all. Why would I divide by $ P(A+) $ when I had to move along this path? Sorry for the mix-up of $A+$ and $ B $..