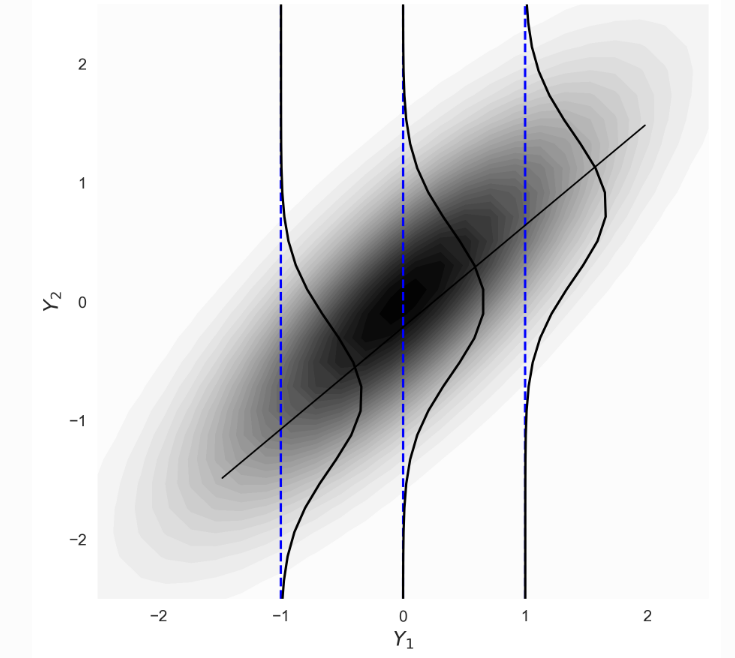
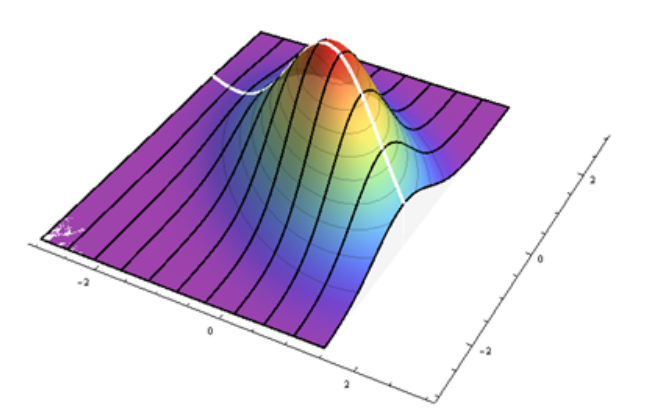
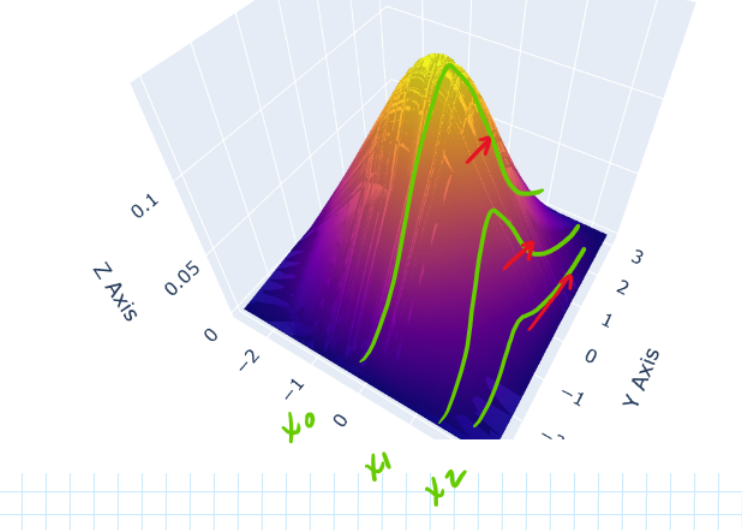
I'm trying to understand the basics of Gaussian Distribution. I struggle to visualice how the variance of the conditional probability of say P (Y|X) changes when X is fixed (given X and Y have a joint gaussian distribution). So, I have two pictures , in picture A from many sources shows that the variances dont change but the mean does. But it seems reasonable to me also to consider the conditional as a cut of de bivariate along one axis but now the variances does change, why is it so? I'm I wrongly thinking Picture B is the conditional?
Thanks for your time.
Picture A
[Picture updated thanks to contribution]
Picture B
Cuts along one variable ,. It seems that the variance (spread of black lines) increases or decreases according to a fixed X.