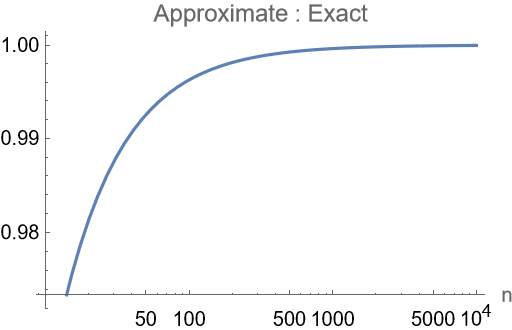
I just want to point out that the standard deviation of $s$ can be well approximated by $SD(s)\approx \sigma/\sqrt{2n}$$\text{SD}(s)\approx \sigma/\sqrt{2n}$ when $n$ is large. The relative error is less than 0.38% for $n=100$ and decreases even further for larger values of $n$.
One way to derive this result applies the asymptotic expansion of the Gamma function to Macro's formula (elsewhere in this thread),
$$\sigma \sqrt{ 1 - \frac{2}{n-1} \cdot \left( \frac{ \Gamma(n/2) }{ \Gamma( \frac{n-1}{2} ) } \right)^2} = \sigma\left(\frac{1}{\sqrt{2n}} + \frac{3}{2^2\sqrt{(2n)^3}} + \frac{15}{2^5\sqrt{(2n)^5}} + \cdots\right)$$
This shows that (at least eventually) the approximation is a little too low. A plot of their ratio shows this is always the case: