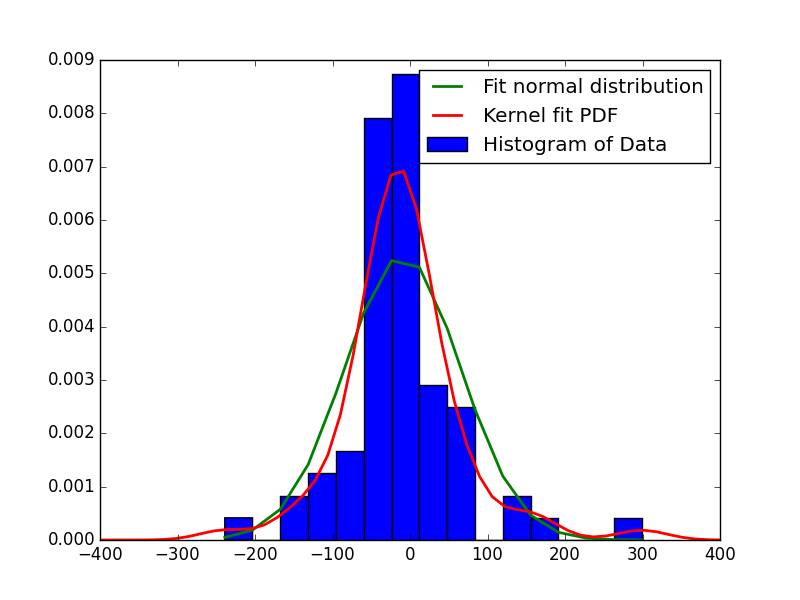
I am not an expert in statistics but I am facing the following problem: I have a random variable that basically arrises as difference of count data. I have 67 points and they don't seem to be normally distributed. Nevertheless I would like to estimate the probability that the underlying random variable takes values larger than, say, 400.
Would it be statistically sound to estimate a PDF using a kernel density estimation and then compute the probability $P(X>400)$ through that estimation? Or could it be a way to go to fit a normal distribution? However, I have to say that testing on normality fails. Please also see the attached figure.
Thanks. :)