7
$\begingroup$

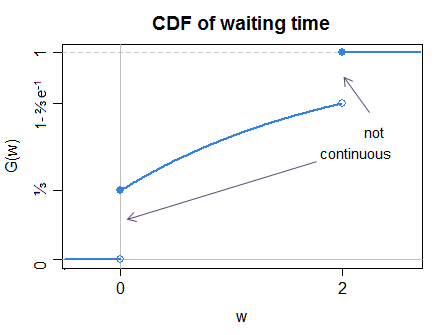
The waiting time, $W$, of a traveler queuing at a taxi rank is distributed according to the cumulative distribution function, $G(w)$, defined by:

$$G(w) = \begin{cases} 0 & \text{ for } w<0,\\ 1 - \left(\frac{2}{3}\right)e^\left(\frac{-w}{2}\right) & \text{ for } 0\le w < 2, \\ 1 & \text{ for } w\ge 2 \end{cases}$$

Is the random variable, $W$, discrete, continuous or mixed?

The solution provided was:

We see the distribution is mixed, with discrete 'atoms' at 0 and 2.

I don't understand the solution. Can I have more details please?

My answer was that the random variable, $W$ is continuous because it represents waiting time and time is a continuous variable. Why is my answer wrong?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
9
$\begingroup$

It's wrong because - as the answer explained - there are discrete atoms at 0 and 2.

By that cdf, you can wait exactly 0 time with positive probability (similarly with 2). Because of that, the waiting time is mixed, not continuous.

Presumably you've been given definitions of all three. How are continuous r.v.s defined?

If it's not immediately clear from the formula, it often helps to draw the cdf:

enter image description here

Improve this answer
$\endgroup$
7
  • $\begingroup$ The given definition of continuous random variable is "Random variables with values that are measured are called continuous random variables". The example given is the maximum temperature reached during a chemical reaction. For discrete random variables, the definition is "quantities that are counted" and the example given is "number of heads in 10 throws of a fair coin". No definition was given for mixed random variables... $\endgroup$
    – mauna
    Commented Jan 4, 2015 at 8:27
  • 2
    $\begingroup$ @mauna: The cdf is discontinuous at 0 and at 2. At 0, it "jumps" from 0 to 1/3, meaning that P[W < 0] = 0 and P[W ≤ 0] = 1/3 -- so P[W = 0] = 1/3. So it's discrete at 0. And similarly at 2, where it "jumps" from 1-2/3e to 1, meaning that P[W < 2] = 1-2/3e and P[W ≤ 2] = 1, so P[W = 2] = 2/3e. But in between 0 and 2, it's continuous, so no specific value has a positive probability. $\endgroup$
    – ruakh
    Commented Jan 4, 2015 at 8:49
  • 5
    $\begingroup$ Both the definitions in your first comment there are wrong (if they're not intended as definitions or characterizations but as examples, they may be fine). You can have a measured random variable that is not continuous, as we see in your question. You can have a discrete random variable that is not a count. Under that (wrong) definition for continuous and no definition for mixed, your answer would be right. In your second comment "for continuous random variable, the probability that it takes on specific value is 0"; that's important- and it explains why the case in the example isn't continuous $\endgroup$
    – Glen_b
    Commented Jan 4, 2015 at 9:18
  • 3
    $\begingroup$ To add to @Glen_b's comment, "the probability that it takes on specific value is 0" must hold for all choices of "specific value" and, as ruakh showed explicitly, the criterion does not hold at $w=0$ and $w=2$. A better definition of discrete random variabe might be that the CDF is a staircase function, for continuous random variable that the CDF is continuous everywhere and differentiable everywhere (except perhaps for a discrete set of points where it is continuous but not differentiable.) $\endgroup$
    – Dilip Sarwate
    Commented Jan 4, 2015 at 15:08
  • 2
    $\begingroup$ @mauna Making all the inequalities in your formula strict will merely leave the cdf undefined at $0$ and $2$, but not eliminate the fact that the cdf has jump discontinuities at $0$ and $2$. For $W$ to be called a continuous random variable, it is necessary that the cdf be continuous everywhere, and your given cdf does not satisfy this criterion.. $\endgroup$
    – Dilip Sarwate
    Commented Jan 4, 2015 at 19:58

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.