6
$\begingroup$

Which of the Gauss-Markov assumptions is violated in this picture?

If all other Gauss-Markov assumptions are satisfied, is the OLS estimator for $\beta_1$ unbiased and consistent? Why?

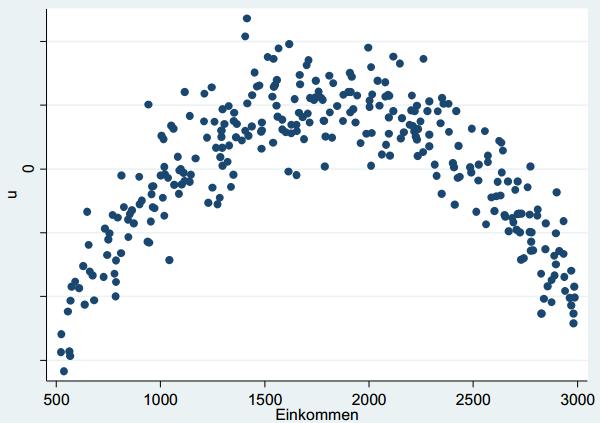
diagramm u and income

In the diagram, u is the error term, Einkommen is income (an explanatory variable).

The model is specified as follows:

$y = \beta_0 + \beta_1 \text{einkommen} + u$

The problem is taken from an exam.

My thoughts (x is einkommen):

the figure shows a quadratic function

the Gauss-Markov assumptions are:
(1) linearity in parameters
(2) random sampling
(3) sampling variation of x (not all the same values)
(4) zero conditional mean E(u|x)=0
(5) homoskedasticity

I think (4) is satisfied, because there are residuals above and below 0

(5) is satisfied, since the variation seems to be constant over all x (3) satisfied , since einkommen is not the same value for all observations (2) random sampling is satisfied, dont ask me why. so only (1) is left, the model is not linear in parameters.

I hope I am not totally wrong with my thoughts.

Improve this question
$\endgroup$
8
  • $\begingroup$ to make that clear: u is the error term in this figure. $\endgroup$
    – Nik
    Commented Jan 15, 2015 at 22:53
  • $\begingroup$ Please state that in your question. When you do, please also explain the relationship between Einkommen and the model: is it a covariate? The dependent variable? The predicted value? A variable not in the model at all? $\endgroup$
    – whuber ♦
    Commented Jan 15, 2015 at 22:54
  • $\begingroup$ i edited my first post $\endgroup$
    – Nik
    Commented Jan 15, 2015 at 22:56
  • 1
    $\begingroup$ Please add the [self-study] tag & read its wiki, then tell us what you understand thus far, & where you are stuck. We will provide hints to help you get unstuck. $\endgroup$
    – gung - Reinstate Monica
    Commented Jan 15, 2015 at 23:04
  • 1
    $\begingroup$ Thank you for your ongoing improvements to the question. You are right to focus on (4): please consider the distinction between "mean" and "conditional mean." You are also correct to point out that (2) simply cannot be verified. Since you have told us that $u$ is the "error term," that implies it was already obtained by a linear model, so you have no basis to reject (1): that is a given (if only implicitly). $\endgroup$
    – whuber ♦
    Commented Jan 16, 2015 at 0:08

4 Answers 4

Reset to default
2
$\begingroup$

There've been a couple answers and none of em have touched on what I thought were the most interesting questions asked, the bias and consistency of misspecified linear models. Since it seems pretty clear from the residuals that the model is misspecified with a quadratic term, let's take a look at what happens to our estimates. I'll leave this in terms of a general misspecification instead of solely a quadratic one for funsies.

Suppose we know an oracle who tells us the generating process for the data is $Y=X \beta +Z \alpha +\epsilon$. However, the model we choose to fit is $Y=X \beta+\epsilon$. Take note that the true model contains extra data in the form of Z and extra parameters in the form of the $\alpha $ term. Now, we could think of Z as being data we were unable to or chose not to collect but we could also think of the Z term as being data we collected and chose not to include in our model (like the situation you are in).

Now the typical parameter estimate is $ \hat{ \beta}=(X^{T}X)^{-1}X^{T}Y$. Biasedness relates to the expectation of our estimate and if we want to have consistency, we need that our bias disappears asymptotically. Keeping that in mind, we look at our expectation: $ E [\hat{ \beta}]=(X^{T}X)^{-1}X^{T}E [Y] = \beta +(X^{T}X)^{-1}X^{T}Z \alpha $.

So, if we misspecify, and alpha is not a column of 0's we end up with estimates which will certainly be biased by a factor of $(X^{T}X)^{-1}X^{T}Z \alpha $. Likewise, since consistency depends on asymptotic unbiasedness and our bias term has no reason to disappear asymptotically, we can expect the parameter estimates to fail to be consistent well.

Improve this answer
$\endgroup$
0
$\begingroup$

It seems that your data does not follow a linear model.

Improve this answer
$\endgroup$
3
  • $\begingroup$ that means, assumption MLR1 (linearity in parameters) is violated. $\endgroup$
    – Nik
    Commented Jan 15, 2015 at 22:50
  • 1
    $\begingroup$ It depends on what you mean by "linear model". Under the typical definition, it isn't clear that this the issue. $\endgroup$
    – gung - Reinstate Monica
    Commented Jan 15, 2015 at 23:06
  • $\begingroup$ with linear i mean the beta - coefficents are linear $\endgroup$
    – Nik
    Commented Jan 15, 2015 at 23:20
0
$\begingroup$

First note that the data look to have a quadratic as opposed to linear relationship. This casts doubt on the linearity assumption:

$$ E[Y\,|\,einkommen] = \beta_{0}+\beta_{1}einkommen$$

My hint to you is this: assume to the contrary that the model is linear, perform the regression (either hypothetically or literally) than check the other assumptions, do they hold?

Extra hint: $E[u]=0$ does not imply $E[u_i|einkommen_i] = 0$ for all $i$.

Improve this answer
$\endgroup$
1
  • $\begingroup$ thanks for your extra hint. now i see, that MLR4 (zero conditional mean: E(u|x)) is violated. $\endgroup$
    – Nik
    Commented Jan 16, 2015 at 10:48
0
$\begingroup$

The main issue is with assumption #5, i.e. homoscedasticity. Your error variance seems to change with income. It's higher in the middle than at the ends.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.