3
$\begingroup$

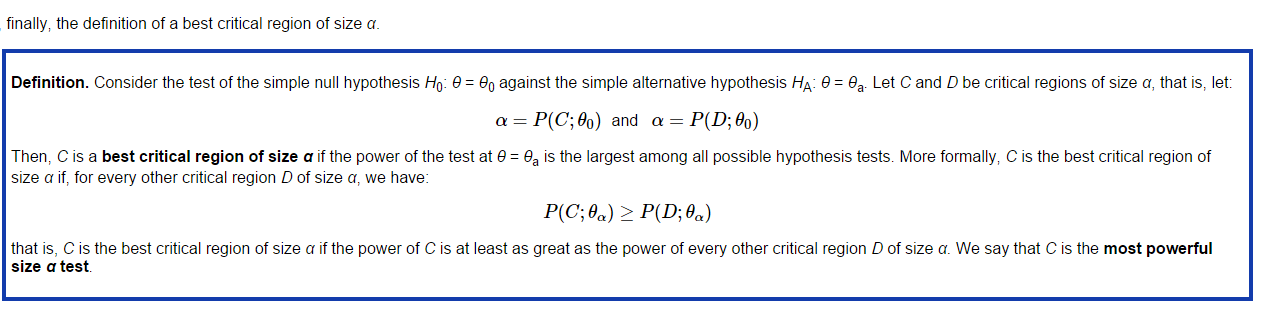
I'm reading an online course and I'm really confused about the Neyman-Pearson lemma. It states

enter image description here

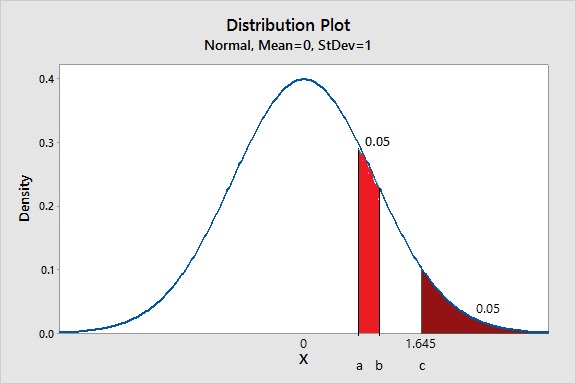
From my understanding, does it mean that critical region could be any region like (a,b) and (c,+00)? enter image description here

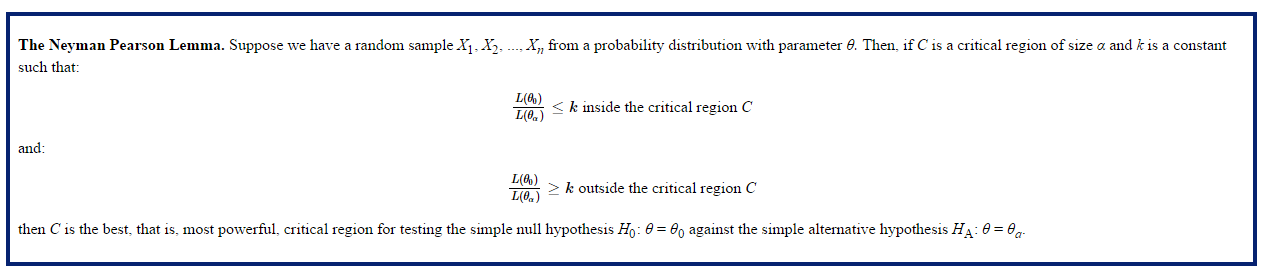
And I'm very confused with most powerful critical region according to its definition enter image description here

what does K mean? Can K be any constant number?

Improve this question
$\endgroup$
2
  • $\begingroup$ It means a constant number, which you need to determine so that the test has the required significance level. $\endgroup$
    – JohnK
    Commented Jan 28, 2016 at 23:23
  • $\begingroup$ I posted an illustrated account of this theory at stats.stackexchange.com/a/130772. $\endgroup$
    – whuber ♦
    Commented Jan 29, 2016 at 0:33

1 Answer 1

Reset to default
2
$\begingroup$

Both of the regions you shaded correspond to $\alpha=0.05$, assuming that your plot represents the null distribution.

The best critical region would be the one of these that would be most likely under the alternative hypothesis. If the alternative hypothesis was $H_a:\mu = C$ for any $C>0$, then the tail critical region would be the best critical region because it would be the critical region with the largest probability under the alternative.

K is a unique constant so that the test has the specified significant level, as the commentator JohnK noted.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.