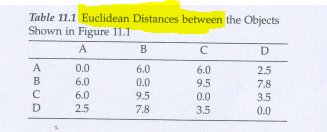
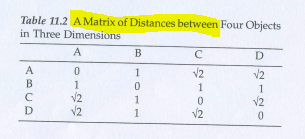
The picture below concerns Multidimensional Scaling (MDS), in which there are two terms " Matrix of distance "(δ) and "euclidean distance"(d). I don't understand the difference between them. Can you explain it?
$\begingroup$
$\endgroup$
1
-
$\begingroup$ See stats.stackexchange.com/a/31291/3277. The input distances should be better called "dissimilarities" (they are not necessary computed from variables, in some studies respondents are directly asked to "estimate" unlikeness between stimuli). The distances resultant in MDS are the ones observed on the fitted map. The "in-between" state between both are called disparities. $\endgroup$– ttnphnsCommented Nov 12, 2016 at 6:50
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
Answered in comments, copied below:
See What is the role of MDS in modern statistics? . The input distances should be better called "dissimilarities" (they are not necessary computed from variables, in some studies respondents are directly asked to "estimate" unlikeness between stimuli). The distances resultant in MDS are the ones observed on the fitted map. The "in-between" state between both are called disparities. – ttnphns
answered Aug 27, 2017 at 13:11