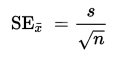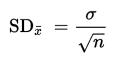Standard error of the mean misunderstanding

Question

I am confused about the second formula below, I would like some intuition here. Please correct me if any of my statements are false.

For the first formula :

It's like saying that by taking the SD of the means of all our samples we get the SEM. This SEM will show us how far away is our "mean of means" (mean of all our sample means) from the TRUE population mean, so this is an estimate for the TRUE mean.

For the second formula :

I don't know what exactly it tries to tell us. But I assume that sampling enough and the calculating the SD of each sample appart and then take the mean of all these sample SDs will get us close to the SD of "the means of means" or with other words , it will get us close enough to the true population SD. What kind of estimate is this here ?

https://en.wikipedia.org/wiki/Standard_error

Answer 1

• The second formula is the standard error of your sample mean. It is the formula you should use whenever possible.
• Unfortunately, you can't use the second formula because you don't know the true standard deviation.
• Instead, you assume the sample standard deviation is unbiased and use it for the true standard deviation.
• Thus, the two formulas are more or less doing the same thing (not exactly statistically, but you may want to interpret them like that)

the SD of the means of all our samples...

I don't agree the wording. You may want to say "the standard error formula I see estimates the variability of my sample mean between samples".

Remember:

The standard error of the mean estimates the variability between samples whereas the standard deviation measures the variability within a single sample.