How to deal with critical value hypothesis test when test statistic is negative?

Question

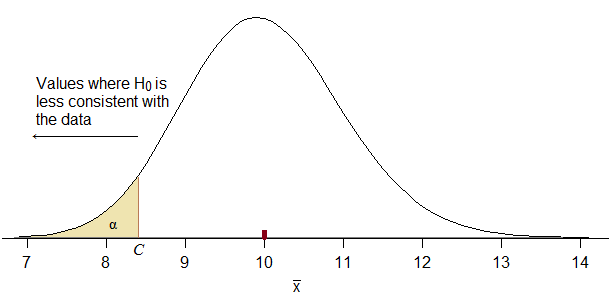
I am solving the following problem:

An electronic tablet producer claims that the batteries on their tablets lasts for 10 hours but from my experience I think it is less than that. It is known that the battery life-span follows an exponential distribution. A random sample of size 100 (supposed to be independent) is tested, for which the mean battery life is found to be 9 hours. Test whether the producer's claim is true at a 5% significance level using the critical value method.

---

My Work

We can model the $i$th tablet battery life-span as $X_i \sim Exp(\lambda)$ where the lifespan is measured in hours. Each $X_i$ has mean $\dfrac{1}{\lambda}$ and standard deviation $\dfrac{1}{\lambda}$. A sensible estimator of $\mu = \dfrac{1}{\lambda}$ is then just $\bar{X}_n$.

The null hypothesis is $H_0 : \mu = 10 = \dfrac{1}{\lambda}$,

and the alternative hypothesis is $H_1 : \mu = \dfrac{1}{\lambda} < 10$.

The appropriate test statistic is $Z = \dfrac{\bar{X}_n - \mu}{\dfrac{\sigma}{\sqrt{n}}} = \dfrac{\bar{X}_n - 10}{\dfrac{10}{\sqrt{100}}}$

Assuming the sample size is large enough for the CLT to apply, we can get our observed test statistic::

$Z = \dfrac{9 - 10}{\dfrac{10}{10}} = -1$

I now calculate the critical value at a 5% level of significance:

$P(z > z_{0.05}) = 0.05$

$\implies 1 - P(z < z_{0.05}) = 0.05$

$\implies 0.95 = P(Z < Z_{0.05})$

$\implies Z_{0.05} = 1.64$

This is my point of confusion: How do I deal with the negative value for $Z$ (the test statistic)? When doing these types of problems, I have always derived a positive value for the test statistic, so I'm unsure of how to proceed.

I would greatly appreciate it if people could please take the time to explain what one does (and why) when they derive a negative value for the test statistic, as done above.

EDIT:

My understanding is that, when hypothesis testing using the critical value method, we are seeking to reject the null hypothesis if it falls inside an arbitrary interval along the tails of the standard normal distribution, based on the indicated level of significance. When we calculate the test statistic, $Z$, we get a value of $-1$. This is the same $Z$ that is used in calculating quantiles: $P(Z > a_\alpha) = \alpha$. The numerical value of $Z$ for quantiles is irrelevant, but the sign (+ or -) IS relevant. Therefore, we have, in this case, that $P(-Z > a_{0.05}) = 0.05$, which implies that $P(Z < -a_{0.05}) = 0.05$. After doing further simple calculations, we find that $P(Z < a_{0.05}) = 0.95$; therefore, $a_{0.05} = 1.64$.

Now, since we want to reject the null hypothesis if the test statistic is on the tails of the standard normal distribution (too unlikely in either direction), we reject values that are $< -1.64$ or $> 1.64$?

Answer 1

Let's consider what we're trying to do at a very basic level.

Here's the actual density of the average lifespan of 100 batteries under the null. It's somewhat skew but will be not-too-terribly approximated by a normal:

If the manufacturer's batteries don't last as long as claimed, we should see a lower average than 10. So we want to reject when our estimate of $\mu$ ($\hat \mu = \bar{x}$) -- i.e. the sample mean -- is sufficiently far below 10 that $\mu=10$ isn't a tenable claim.

Consequently, our rejection rule must correspond to something of the form "reject for $\hat \mu \leq C$" for some suitable choice of $C$. If we don't get that we clearly made a mistake.

How do we choose $C$? We want $C$ to be as high as possible (i.e. close to $10$ from below, so that we maximize power) while keeping the probability of falling in the rejection region when $H_0$ is true to be no more than $\alpha$:

When you use a normal approximation for this, you still want it to correspond to rejecting small values of $\bar{x}$. If you don't do that, it won't correspond to your stated alternative hypothesis (potentially leaving you in the silly position of accusing the manufacturer of shorter battery life when maybe it's actually longer).

Can you figure out what the $Z_C$ value would be below which $\alpha$ of the probability will lay? (under $H_0$, naturally)

(If you use a calculation approach that yields a $Z_C$ that doesn't look qualitatively like the diagram, you cannot be using the right one. Instead just do the basic calculation the diagram indicates. This is the sort of diagram you should have been drawing for us -- such diagrams are crucial to avoiding errors. I really don't know how they can be letting you try to answer questions like this without insisting you draw a diagram every time.)

What rejection region for $\bar{x}$ would it imply?

(If you use a normal approximation, the actual type I error rate for a nominal 5% test turns out to be 4.35%, which is perhaps a bit further out than many people would hope, but that's hardly the big issue here -- much more important is figuring out the right direction for rejecting the null)

Answer 2

Pointer, I'm a statistical amateur compared to others here, but I think my lack of experience might help me understand what you're having difficulty with.

Negative values are perfectly OK in the test statistic here since your reference distribution can take negative values - as you're using the CLT, your null distribution is the standard normal distribution, which is symmetric around the y-axis (look it up on Wikipedia if you're not sure what I mean). The other possible source of confusion (if you're already quite familiar with the standard normal) is that you might have only needed two-sided tests up to this point. When doing a two-sided test with a symmetric distribution, much of the time people just use the absolute value of the test-statistic and halve the significance level (many people code it in R this way).

Last, here, since you have a one-sided alternative hypothesis, and since the Z-transformed statistic essentially puts your hypothesized mean (10) at 0 (the "minus mu" in the equation), if you think about it, you'll see that your alternative hypothesis would have no chance whatsoever of being true, even before looking up Z(0.05), if your test statistic were positive.

To other, more experienced statisticians here, please correct me if I've given any misinformation (and if I have, many apologies!). I'm trying to learn myself!

Answer 3

Based on your $H_0: \mu = 10$ and $H_1: \mu < 10$, the $H_0$ will be rejected if sample mean is < a specified number. So it should be:

$\Pr(z < z_{0.05}) = 0.05$

$\implies Z_{0.05} = -1.64$

It means if sample z value is < -1.64, then reject $H_0$ at $\alpha = 0.05$ level (one-side test). Your z is -1 > - 1.64, so there is no enough evidence to reject $H_0$ that $\mu = 10$.