1
$\begingroup$

My question is whether it is plausible to have "Rate Ratio" showing an increased risk while "Odds Ratio" (based on the same data) showing decreased risk?

I have an example in this article: http://jamanetwork.com/journals/jamapediatrics/fullarticle/203581

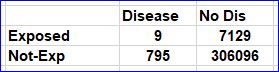
The article reported a rate ratio of 2.05 (95%C.I.= 1.06-3.97) for "any use of erythromycin" (Table 2 in the article). Constructing the 2x2 table, gives the following:

2 by 2 table

Based on this 2x2 table, odds ratio = 0.49 (95%C.I.= 0.25-0.94).

How can we explain this conflict?

Thank you.

Improve this question
$\endgroup$
2
  • $\begingroup$ Do you suppose it might be a coincidence that $1/2.05 = 0.49$? And that $1/1.06 = 0.94$ and $1/3.94 = 0.25$? $\endgroup$
    – whuber ♦
    Commented Sep 1, 2017 at 22:00
  • $\begingroup$ Thank you. I thought about this but I was confused by the authors explicitly stating that "exposure to erythromycin ... was associated with a 2-fold increased risk of pyloric stenosis (adjusted rate ratio, 2.05; 95% confidence interval, 1.06-3.97) (Table 2)." Is it a mistake in their reporting? $\endgroup$
    – Anna
    Commented Sep 1, 2017 at 22:12

1 Answer 1

Reset to default
3
$\begingroup$

You and the article are just looking at reciprocal ratios.

$$(9/7129)/(795/306096) = 0.49$$ $$ (795/306096)/(9/7129) = 2.06$$

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ Thank you. I was confused by the authors explicitly stating that "exposure to erythromycin ... was associated with a 2-fold increased risk of pyloric stenosis (adjusted rate ratio, 2.05; 95% confidence interval, 1.06-3.97) (Table 2)." Is it a mistake in their reporting? $\endgroup$
    – Anna
    Commented Sep 1, 2017 at 22:10

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.