5
$\begingroup$

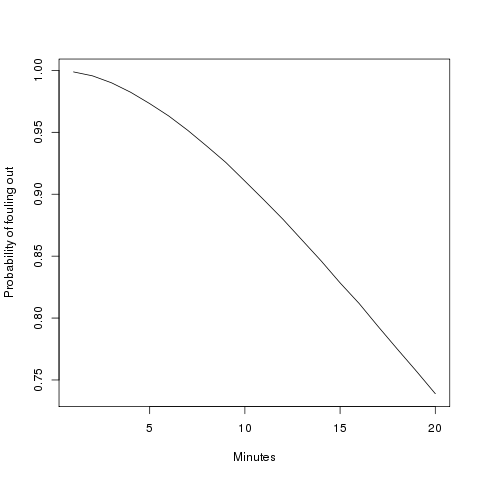
Kevin Durant is a world-class basketball player. He averages roughly 2 fouls for every 40 minutes of game play.

Per NBA rules, you must stop playing after picking up 6 fouls. Supposing Kevin Durant has 4 fouls with 20 minutes remaining in a game:

a) if he tries to play all 20 remaining minutes, what are the odds he acquires two more fouls and has to leave the game?

b) if he were to sit on the bench for 5 minutes (leaving 15 remaining), then what would the odds be?

c) if not 5, is there an ideal number for B in generating a greater likelihood of attaining more minutes than A?

Improve this question
$\endgroup$
12
  • 4
    $\begingroup$ Also, is this homework? $\endgroup$
    – Macro
    Commented Jun 18, 2012 at 21:48
  • 2
    $\begingroup$ @Macro For the purposes of this question, any minute is as likely to draw a foul as the next. Also, it's not homework - I'm having a debate with friends and I'm not a mathematician. $\endgroup$
    – Samuel Hulick
    Commented Jun 18, 2012 at 21:58
  • 2
    $\begingroup$ Under mild assumptions (that I won't state :)), benching a player with foul trouble strictly decreases the expected number of minutes the player plays; the intuition is that you are voluntarily imposing on yourself the very penalty that you are trying to avoid. All you are doing is shifting their expected foul-out time distribution by the amount of time you bench them. But NBA coaches are willing to let their leads evaporate or their opponents blow them out because god forbid they let their star player foul out. $\endgroup$
    – guy
    Commented Jun 18, 2012 at 23:14
  • 3
    $\begingroup$ I think the reason the practice persists is because a lot of people think that not all minutes in basketball are created equal, and so it is better to "save" your stars for the end of the game. There is some empirical evidence that they are correct, but I would argue this is sort of a self-fulfilling prophecy. $\endgroup$
    – guy
    Commented Jun 18, 2012 at 23:18
  • 1
    $\begingroup$ @Guy The assumption coaches are making by benching players is that being in foul trouble restricts the way you play. You've got to defend a little more cautiously to avoid the risk of further fouls. Benching a star gives them a rest and brings on a bench player who knows they will play few minutes anyway, so can defend at full intensity knowing that a foul won't have a huge impact. A bench player might play as well as a star in foul trouble and benching ensures all star minutes are played at high intensity. That is the theory anyway. Of course, the OP has asked us to ignore all this... $\endgroup$
    – Bogdanovist
    Commented Jun 18, 2012 at 23:37

2 Answers 2

Reset to default
2
$\begingroup$

There has actually been some research on this(!). Here's a paper by Maymin et al. (2012), (SSRN Link). They actually look at win-loss statistics instead of just trying to maximize the number of minutes a player plays. They argue that:

  • Getting into foul trouble causes a player to play measurably worse, and so
  • It's rational to yank a player with Q+1 fouls (where Q is the present quarter)

There's a shorter piece of Slate, which is where I found the original reference. Enjoy!

Improve this answer
$\endgroup$
5
$\begingroup$

If we stick rigidly to the assumption "any minute is as likely to draw a foul as the next" then we can model this simply as a Poisson process. The results are shown in the figure below. Probability of Durant making 2 more fouls

The vertical axis shows the probability that Durant has not been fouled out after playing the number of minutes shown on the horizontal axis.

Reading off the numbers at each time asked, there is a 74% probability of Durant going the distance if he attempts to play all 20 minutes. If he is benched for 5 minutes, he will only be able to play for a maximum of 15 minutes and the probability he will not foul out in that time increases to 83%.

As for part c), there is no trade off involved. Durant should never be benched if the goal is to maximise the number of minutes played.

For what it's worth I don't think that the assumption of fouls being a Poisson process is true, but then the result of the analysis would entirely depend of what other assumptions are being made.

Improve this answer
$\endgroup$
8
  • $\begingroup$ Thank you! The "Minutes" axis is "minutes rested", not "minutes played", correct? $\endgroup$
    – Samuel Hulick
    Commented Jun 19, 2012 at 3:14
  • $\begingroup$ @SamuelHulick No No! The minutes axis is minutes played before fouling out. This doesn't have to be consecutive minutes, so if he plays for 5, gets benched for 5, then plays another 5 he has played 10 minutes in total, then reading off from the graph he will have 92% chance of not being fouled out in the period. $\endgroup$
    – Bogdanovist
    Commented Jun 19, 2012 at 3:16
  • 1
    $\begingroup$ There was some discussion on SportCenter this morning regarding the Thunder's decision to bench Durant during the last 5 minutes of the 3rd quarter (after a 4th foul) where a 9 point lead evaporated and turned into a 2 point deficit that was crucial toward Miami's win. $\endgroup$
    – Michael R. Chernick
    Commented Jun 19, 2012 at 12:38
  • 1
    $\begingroup$ @MichaelChernick I completely agree that a Poisson model is a terrible model for this situation. The question asked us to make that assumption though, so that's what I presented. I don't think the answer sheds any light on the real world question of who should be benched when. $\endgroup$
    – Bogdanovist
    Commented Jun 19, 2012 at 22:38
  • 1
    $\begingroup$ @MichaelChernick Sorry yes you're right, the original question was ambiguous. I forgot that it was in a comment that the OP specified "For the purposes of this question, any minute is as likely to draw a foul as the next". If that is considered as part of the question then the assumptions are all made. It's clear that this describes a Poisson process, even if the OP didn't use the explicit technical term. $\endgroup$
    – Bogdanovist
    Commented Jun 20, 2012 at 3:48

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.