0
$\begingroup$

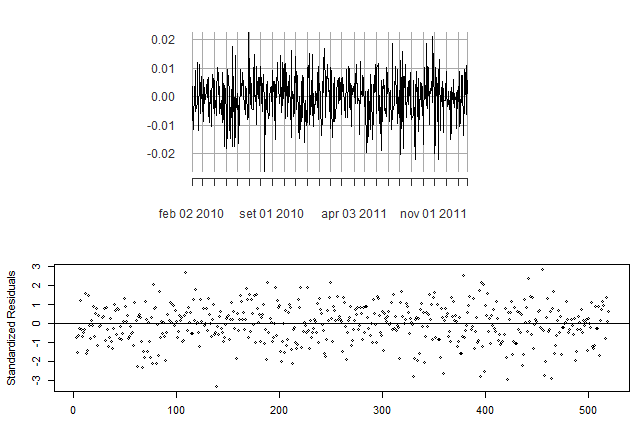
I'm not sure about the presence of a GARCH effect in this first differenced series. What do you suggest? I've fitted an ARIMA(0,1,0), so the original series is a random walk. I'm working with eurusd exchange rates.

enter image description here

Here you can found the initial plots https://ibb.co/m80N8H and here the squadre residuals of ARIMA(0,1,0) plus Mcleod Li test. https://ibb.co/dOgdbS . I've tried also an ARIMA(3,1,0) but it seems the original series is a random walk.

Improve this question
$\endgroup$
8
  • 3
    $\begingroup$ There is not enough information to answer your question. But why don't you run an ARCH-LM test which is specifically suited for identifying presence of ARCH effects? $\endgroup$
    – Richard Hardy
    Commented Feb 21, 2018 at 18:47
  • $\begingroup$ I've add other plots above. However, I ve tried the test but I have problem to interpret it. The test must be run on the differenced series or on its residuals $\endgroup$
    – Kimira
    Commented Feb 22, 2018 at 10:10
  • $\begingroup$ You run the test directly on the data you are examining. If you care about the original series, run it on the original series. If you care about residuals, run it on residuals. The plots in the second link indicate no ARCH patterns. $\endgroup$
    – Richard Hardy
    Commented Feb 22, 2018 at 10:22
  • $\begingroup$ I never understood this aspect. Summing up, there is no serial correlation in my squared residuals, so there is no Arch effect. But there is no arch effect in the residuals or in the differenced time series? What's the procedure that i have to follow? 1. I fitted an ARIMA(0,1,0) because data seems to be a random walk 2. Squared residuals have no correlation so no arch effect. What s the next step? Thanks very much $\endgroup$
    – Kimira
    Commented Feb 22, 2018 at 10:37
  • $\begingroup$ Next step? If there are no GARCH effects, then there is no need for a GARCH model. More precisely, there is no need to add a GARCH-type conditional variance equation to your current model. Keep the model you have now. $\endgroup$
    – Richard Hardy
    Commented Feb 22, 2018 at 13:40

1 Answer 1

Reset to default
0
$\begingroup$

For the residuals plotting there seems to be a pattern. Have you tried higher order of AR and MA? A first glance for Arch effect is testing non correlation over squared residuals (ljung-box)

Improve this answer
$\endgroup$
2
  • $\begingroup$ I put above other plots. The squared residuals seems to go well, do you think so? $\endgroup$
    – Kimira
    Commented Feb 22, 2018 at 10:07
  • $\begingroup$ What do you mean in your second sentence? $\endgroup$
    – Richard Hardy
    Commented Feb 22, 2018 at 10:25

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.