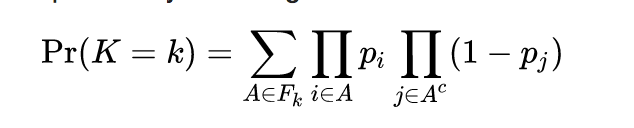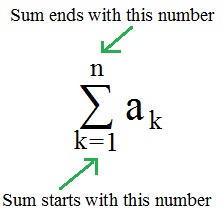$i \in A$: $A$ is a particular collection of indices for which the event occurred,. For example, if we have five trials, the "event" occurred on three of them, and the first, fourth, and fifth trials were the ones on which the three events occurred, $A = \{1, 4, 5\}$. We multiply the associated probabilities $p_i$ together. $i$ refers to the individual indices within $A$.
$j \in A^c$: $A^c$ is the complement of $A$, i.e., the collection of indices for which the event did not occur, e.g., to continue the previous example, if we have five trials and $A = \{1,4,5\}$, $A^c = \{2, 3\}$. We multiply the associated probabilities of the event not occurring $(1-p_j)$ together. $j$ refers to the individual indices within $A^c$.
$A \in F_k$. If $K=k$, that means $k$ events occurred. However, which $k$ is unknown, so you have to sum over all possible arrangements of the $k$ events occurring in the $n$ total trials. $F_k$ refers to all possible arrangements of indices for which $k$ events (out of $n$ trials) occurred.
The number of indices in $A$ and the number of indices in $A^c$ must add up to the total number of trials $n$.
For example, say $n=3$ and $k=2$:
$$F_k = \{(1,2), (1,3), (2,3)\}$$
as these are all the ways two out of the three trials can have events - the first and second, the first and third, or the second and third.
$$A \in F_k: A = (1,2) \text{ or } A = (1,3) \text{ or } A = (2,3)$$
$$i \in A: \text{ if }A = (1,2), \text{ then } i \text{ iterates over } (1,2)$$
$$j \in A^c: \text{ if }A = (1,2), \text{ then } A^c = 3, \text{ so } j = \text{ only }3$$
The outer sum iterates over the (three in this case) elements of $F_k$. The $i$ loop iterates over the elements of whichever $A$ the outer sum is at at the moment, and the $j$ loop iterates over the elements of $A^c$, which are defined as whatever isn't in $A$.