1
$\begingroup$

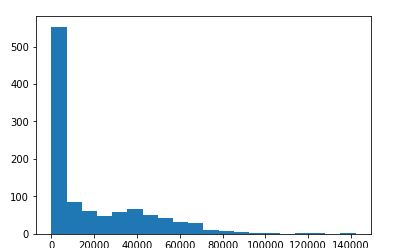
I have two not normal distributions (~1k samples in each), looks like exponential: enter image description here

So, I need to check its means, that's why I have following questions:

  1. The easiest way to do it - is to use Mann-Whitney criteria?
  2. How to check, is it really exponential distribution? Kolmogorov-Smirnov? But it suits only for standard distr?
  3. And is it possible to use CLT for comparison of means: to generate a lot of means using bootstrap from two distr. Accordingly to CLT, means' distributions have to be normal and it becomes possible to use Students criterias. (Presuming, that it is a rather silly question...)
Improve this question
$\endgroup$
1
  • $\begingroup$ the CLT is the easiest way to do it, the assumption is that the mean of 1000 samples is approximately normally distributed. then you calculate the standard error etc, just using the variance of the individual samples. ( so you only need to bootstrap if you want to double check that over 1000 samples, the distribution is normal, not to calculate the standard error) $\endgroup$
    – seanv507
    Commented Jan 31, 2019 at 14:09

1 Answer 1

Reset to default
1
$\begingroup$

Are you sure you want to compare the means?

  1. Mann-Whitney is the non-parametric version of the t-test when the assumptions of the t-test do not hold, you should check the assumptions before you needlessly underpower your test with the MW test.
  2. KS test can be used to compare similarity of two distributions, however be advised that the test is very sensitive with big sample sizes.
  3. You're not going to use the CLT, but the CLT allows you to compare the two means using confidence intervals, so testing for differnce.
Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.