Marginal derivation from joint pdf

Question

I have a uniform prior f(Θ) ~ U(4,10) and a uniform 'observation' model f(X|Θ) ~ U(θ-1, θ+1). Their joint pdf is f(X,Θ)=1/12 for 4 < θ < 10 and (θ-1)< x <(θ+1)  and 0 otherwise.

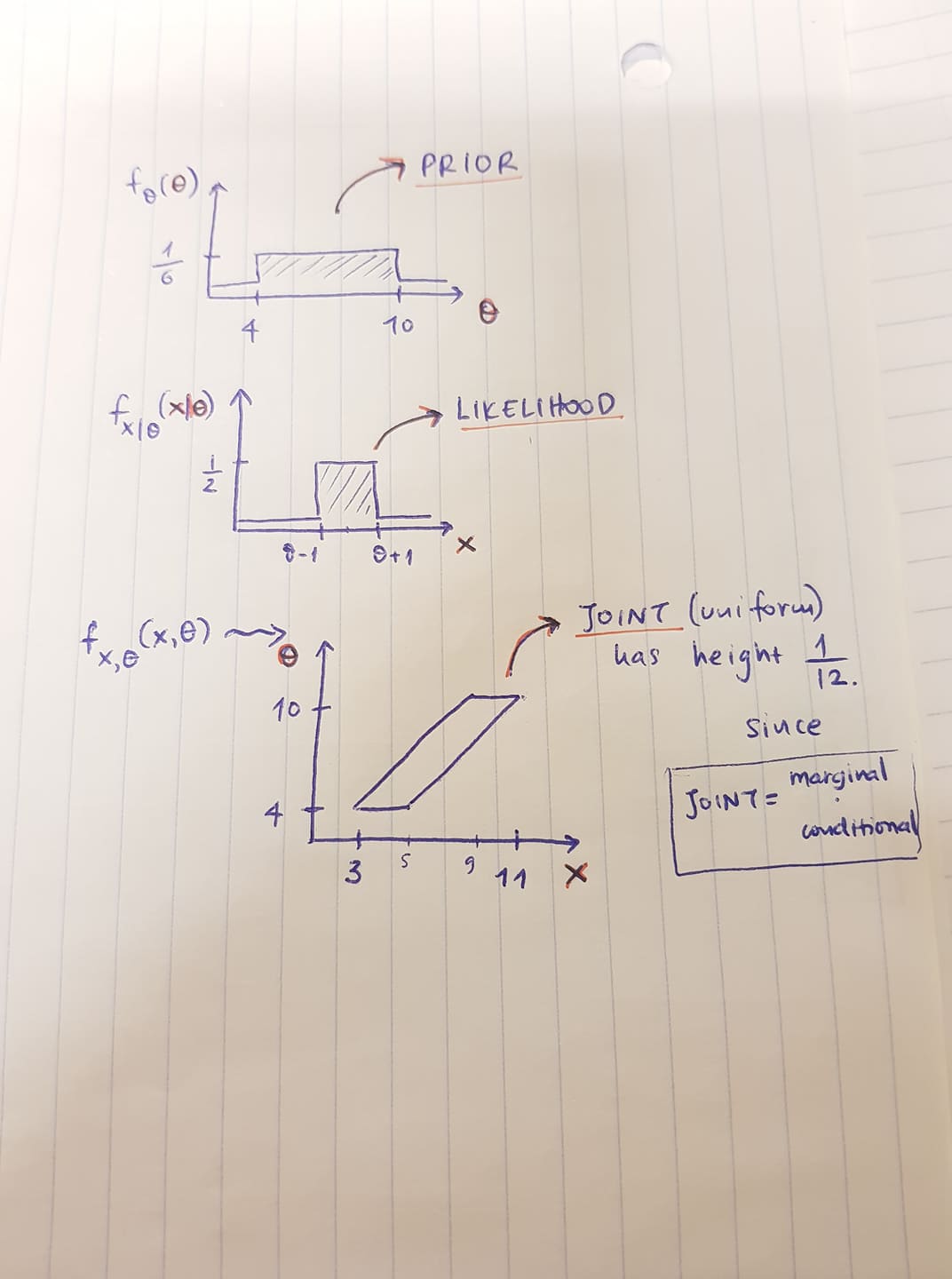
If I compute the marginal f(Θ) as the integral of joint over x (from x=3 to x=11), the answer I get is 1/4. But this is not even a pdf since the area under the curve is not 1.

Samewise, the marginal f(x) is 1/2 but again this is not correct. Am I doing something wrong in the integral bounds?

Also, if the marginal f(X) is uniform that means that eg. x=3(that can happen only for θ=4) has the same probability to occur as x=7 (that can happen for θ=6, θ=7, θ=8). Does that make sense? I got confused in general..

Answer 1

For $f(\theta)$, you fix the $\theta$ and integrate the joint $\forall x$: $$f(\theta)=\int_{-\infty}^\infty f(x,\theta)dx=\int_{\theta-1}^{\theta+1}\frac{1}{12}dx=\frac{1}{6}$$ Here the limits are chosen for each fixed $\theta$. For a given $\theta$, joint PDF is non-zero only in $[\theta-1,\theta+1]$. For visualizing this, draw a line for an arbitrary $\theta$ value, say $\theta=5$, and note the intersection points of the line with the joint PDF's area of support.

For $f(x)$, you fix $x$ and try to formulate where the joint is non-zero. For example, for $3\leq x\leq 5$, the integral bounds for $\theta$ are from $4$ to $x+1$, which is the y-value of the intersection point of the vertical line (for some $x$ in $[3,5]$) and the line passing through points $(3,4)$, $(9,10)$, i.e. $\theta=x+1$. You'll need to consider three regions for $x$, and the resulting PDF won't be uniform.