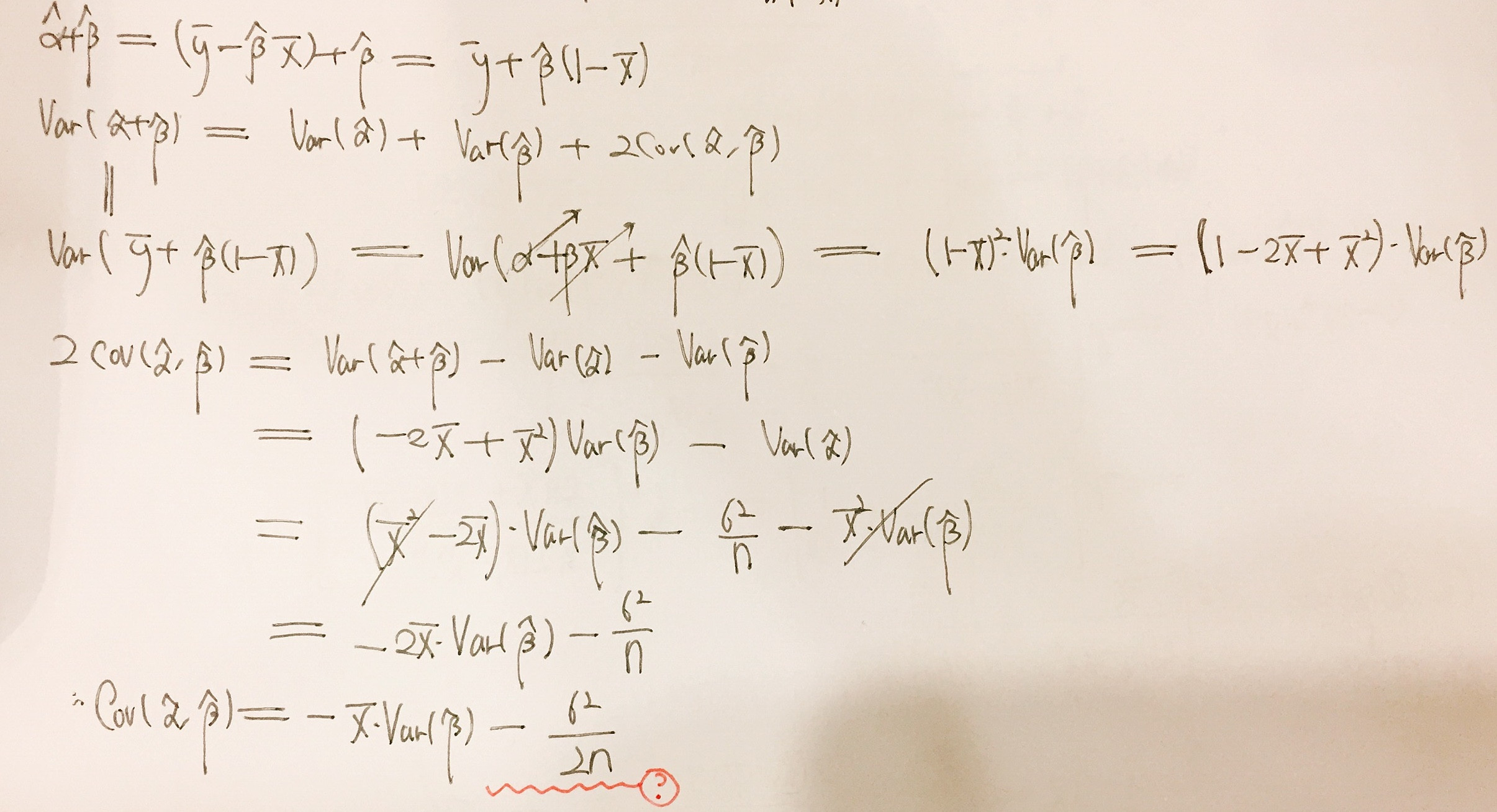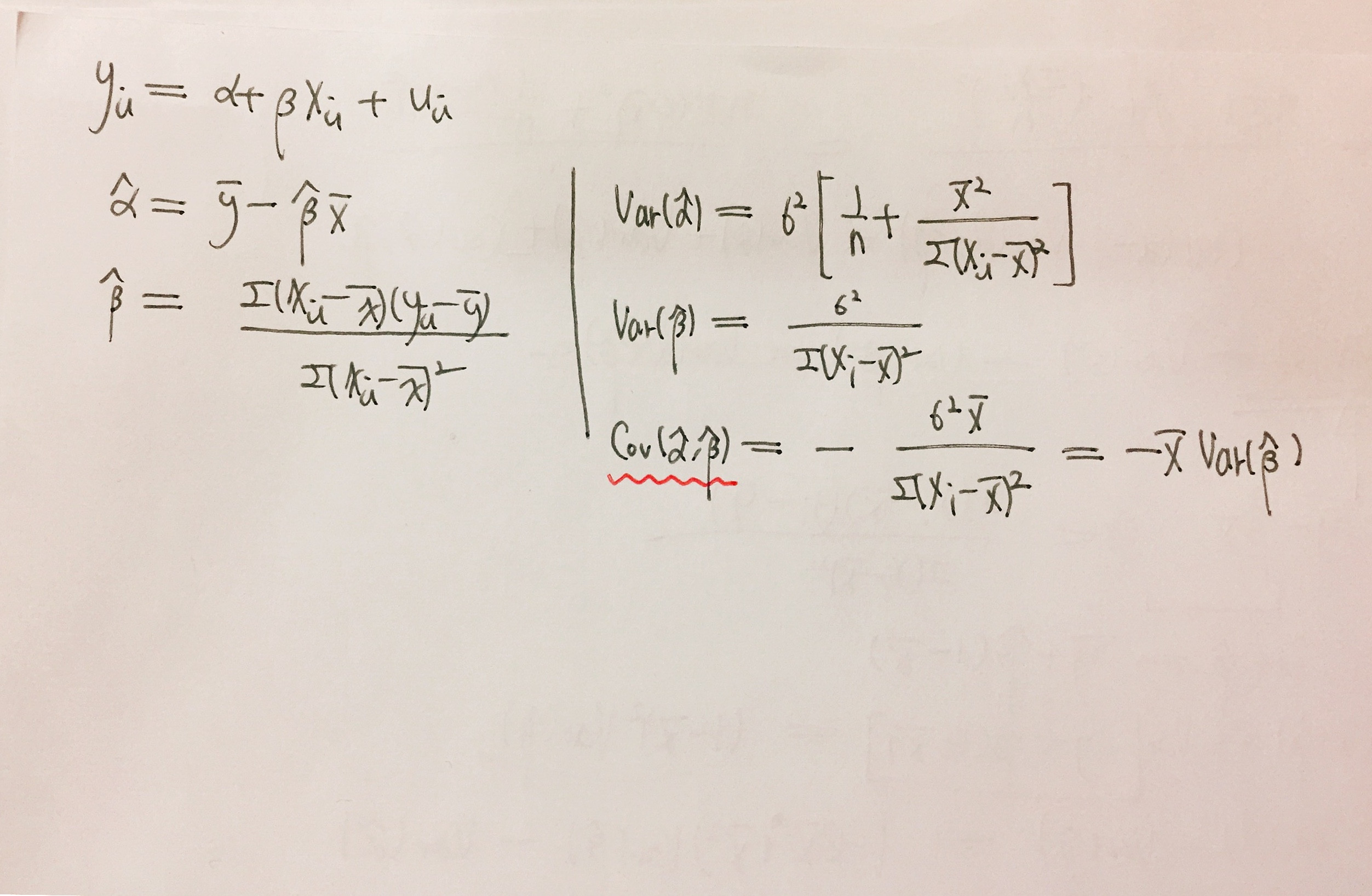Covariance between estimates of slope and intercept [duplicate]

Ask Question

Asked 5 years, 8 months ago

Modified 4 years, 3 months ago

Viewed 5k times

I wonder why the covariance between estimates of slope ($\hat{\alpha}$) and intercept ($\hat{\beta}$) is $-\bar{X}\times Var(\hat{\beta})$.

How can I derive this solution by not using matrix?

I add a new picture of my solving steps. I tried to use the rule of variance - which is $Var(x+y)=var(x)+var(y)+cov(x,y)$ - to derive covariance of $\hat{\alpha}$ and $\hat{\beta}$. But as you can see, the final solution I got is something wrong. I can't understand why '$-\sigma^2/2n$' adds to $-\bar{X}\times Var(\hat{\beta})$.

Can you plz help me find the stage in which I made mistake...?

develarist

4,0491 gold badge23 silver badges58 bronze badges

asked Apr 16, 2019 at 10:53

Dayeon

291 silver badge4 bronze badges

2

$\begingroup$ First show that $\mbox{Cov}(\bar y,\hat\beta)=0$. $\endgroup$
– Jarle Tufto
Commented Apr 16, 2019 at 10:56
1

$\begingroup$ See: stats.stackexchange.com/questions/127316/… $\endgroup$
– kjetil b halvorsen ♦
Commented Apr 16, 2019 at 11:37
$\begingroup$ This question has been in various forms on this site. Like stats.stackexchange.com/questions/68151/…, stats.stackexchange.com/questions/171125/…. $\endgroup$
– StubbornAtom
Commented Apr 16, 2019 at 12:15
1

$\begingroup$ Please explain us why none of the links can help you? $\endgroup$
– kjetil b halvorsen ♦
Commented Apr 16, 2019 at 12:52
1

$\begingroup$ ![Found a more elegant way of proving the same.Hope it helps.](i.sstatic.net/bjEJy.jpg) Found a more elegant way of proving the same.Hope it helps. $\endgroup$
– Paritosh
Commented Sep 6, 2020 at 12:42

| Show 3 more comments

0