Question
I have been struggling to convince myself that SD (standard deviation) is about variation or dispersion.
Standard deviation From Wikipedia, the free encyclopedia
In statistics, the standard deviation is a measure of the amount of variation or dispersion of a set of values.
Is it really an appropriate claim to say average distance from the mean is measure of variation or dispersion? It can be, with specific data sets, but is it really appropriate to say so in general?
As stated in Is standard deviation a proper measure of dispersion of data ?, it seems claiming SD as measure for variation or dispersion could be questionable without cautions in what situations it can be.
Standard deviation has become the most widely used measure of dispersion of data. A measure of dispersion can, in the true sense, be regarded as the proper measure of dispersion if the measure is based on the deviations between all pairs of data.
Standard deviation is, however, not based on the deviations between all pairs of data. Accordingly, the perfectness of standard deviation, as measure of dispersion, is questionable. Therefore, the question is " Is standard deviation a proper measure of dispersion of data ? "
I have not been able to understand how is standard deviation, which is not based on the deviations between all pairs of data, a proper measure of dispersion? The explanation is not as clear as to convince that standard deviation, is a proper measure of dispersion.
Is using the word standard appropriate as well? I suppose if each distance is divided by SD, it could be said as standardized, but what is SD itself is standardizing?
If I follow this Standardization explanation:
In statistics, standardization is the process of putting different variables on the same scale. This process allows you to compare scores between different types of variables.
If there are two data sets X and Y, and SD always makes X and Y comparable on the same meaningful scale/unit, then I believe it is. But is it so?
References
Pearson made up this term in 1894 paper "On the dissection of asymmetrical frequency-curves", here's the pdf.

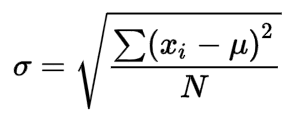
=:signalling that what is on the left is just a name and what is on the right is the machinery that does the work. Many tangles can arise if you take "dispersion" to be a pre-defined property that can only be made precise in one way. As Mosteller and Tukey comment more generally (Data Analysis and Regression) we have many vague concepts in statistics that can be made precise in different ways. $\endgroup$