I have a logit model that comes up with a number between 0 and 1 for many cases, but how can we interprete this?
Lets take a case with a logit of 0.20
Can we assert that there is 20% probability that a case belongs to group B vs group A?
is that the correct way of interpreting the logit value?
The logit $L$ of a probability $p$ is defined as
$$L = \ln\frac{p}{1-p}$$
The term $\frac{p}{1-p}$ is called odds. The natural logarithm of the odds is known as log-odds or logit.
The inverse function is
$$p = \frac{1}{1+e^{-L}}$$
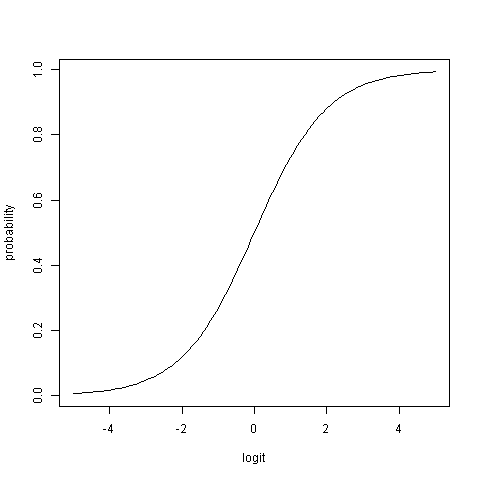
Probabilities range from zero to one, i.e., $p\in[0,1]$, whereas logits can be any real number ($\mathbb{R}$, from minus infinity to infinity; $L\in (-\infty,\infty)$).
A probability of $0.5$ corresponds to a logit of $0$. Negative logit values indicate probabilities smaller than $0.5$, positive logits indicate probabilities greater than $0.5$. The relationship is symmetrical: Logits of $-0.2$ and $0.2$ correspond to probabilities of $0.45$ and $0.55$, respectively. Note: The absolute distance to $0.5$ is identical for both probabilities.
This graph shows the non-linear relationship between logits and probabilities:
The answer to your question is: There is a probability of about $0.55$ that a case belongs to group B.
To add a more modern (but not very deep) perspective, consider how it's used in deep learning (ha, pun intended...):
logit is referred to the output of a function (e.g. a Neural Net) just before it's normalization (which we usually use the softmax). This is also known as the code. So if for label $y$ we have score $f_y(x)$ then the logit is:
$$ logit = \log \left( \frac{ e^{f_y(x)} }{Z} \right) = score = f_y(x)$$
Where $Z$ is the standard partition function. By the way, this is all over the place in the pytorch and tensorflow documentation.
So you can interpret it as:
the (unnormalized) score for a label or (functional confidence) for a specific class/label.
One of the many references: https://stackoverflow.com/questions/41455101/what-is-the-meaning-of-the-word-logits-in-tensorflow
Could you maybe specify your model and give a screenshot of the output, then I could give you an detailed answer, but as a first try.... you may want to check out also the following examples on these websites:
http://www.ats.ucla.edu/stat/stata/seminars/stata_logistic/default.htm
http://www.ats.ucla.edu/stat/stata/dae/logit.htm
http://www.ats.ucla.edu/stat/stata/faq/oratio.htm
http://www.ats.ucla.edu/stat/mult_pkg/faq/general/odds_ratio.htm
so if the coefficient is 0.2 it depends on the variable, I guess you have a dummy, which is e.g. 0 for group B and 1 for group A?
odds ratio is given by: $OR = e^b$
so in your case: $e^{70.20}$
This would be the odds ratio of your group variable corresponding to your reference group.
