Significance test testing whether one variance is larger than the other

Question

I feel like I am missing a key link and this tasks is quite easy: I am given the following averages and standard deviations:

$\bar{x}_1 = 971$, $s_1 = 42$, $n_1 = 1000$

$\bar{x}_2 = 983.9$, $s_2 = 60.18$, $n_2 = 10$

For the second dataset, I have all observations.

What is an appropriate test to check whether $\sigma_1$ is larger than $\sigma_2$? Of course I stumbled upon the F-Test, but as far as I understood it, it can only handle testing whether variances are equal or not; not whether one is larger than the other. A friend of mine used Chi-Square, but I do not see how Chi-Square would be applicable here.

Thank you and best Paul

Answer 1

Assuming normal data, under $H_0: \sigma_2^2/\sigma_1^2, $ the ratio $F =s_2^2/s_1^2 = 60.18^2/42^2 = 2.053 \sim \mathsf{F}(9,999).$

For a one-sided test against $H_a: \sigma_2^2/\sigma_1^2 >1,$ one rejects if the F-statistic exceeds the the 95th percentile $1.8892$ of $\mathsf{F}(9,999),$ called the critical value. So you do (just barely) reject at the 5% level of significance. [For a two-sided test against $H_a: \sigma_2^2/\sigma_1^2$ the critical values would be at $0.299$ and $2.126,$ so you would not reject against the 2-sided alternative at the 5% level.]

qf(0.95,9,999)
[1] 1.889236

qf(c(.025,0.975),9,999)
[1] 0.299400 2.126391

In terms of P-values, the P-value for the 1-sided test is $0.031 < 0.05 = 5\%$ so you'd reject at the 5% level. [The P-value of the 2-sided test would be double the P-value of the 1-sided test.]

1 - pf(2.053, 9, 999) 
[1] 0.0310929

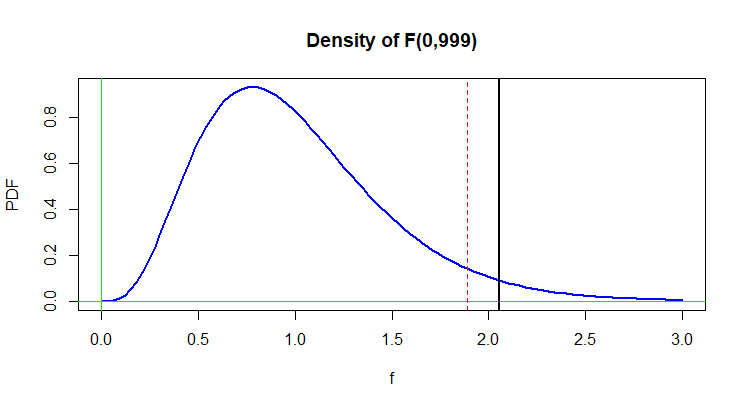
The figure below shows the density function of $\mathsf{F}(9,999)$ along with the observed value of $F$ (solid black vertical line) and the one-sided critical value (dotted red).

Note: Traditionally, the larger sample variance is put in the numerator of the F-statistic because many printed tables of F distributions do not give lower tail probabilities. (Of course, most software programs give probabilities in both tails.)