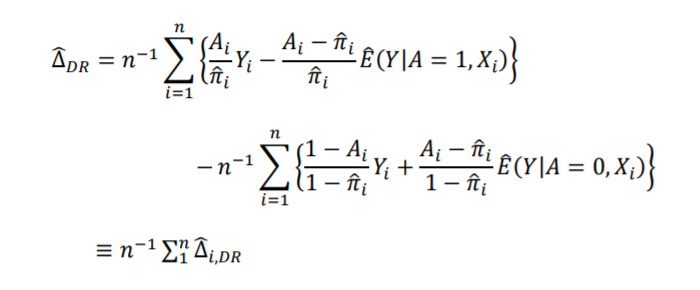How would one convert the ATE Average Treatment Effect from a doubly robust model into Odds Ratio? Or is it better to discuss this by converting into risk ratio? If so, how is this done.
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
3
If your outcome is binary, this is straightforward by noting that that the estimator is the difference between two counterfactual probabilities, and the odds ratio is just a ratio of the odds instead of of the probabilities. So, if
$$\hat{P}(Y^1=1) = n^{-1}\sum_{i=1}^n \left\{ \frac{A_i}{\hat{\pi}_i} Y_i - \frac{A_i - \hat{\pi}_i}{\hat{\pi}_i}\hat{E}(Y|A=1,X_i) \right\}$$ and $$\hat{P}(Y^0=1) = n^{-1}\sum_{i=1}^n \left\{ \frac{1-A_i}{1-\hat{\pi}_i} Y_i - \frac{A_i - \hat{\pi}_i}{1-\hat{\pi}_i}\hat{E}(Y|A=0,X_i) \right\}$$
and we know $\text{odds}(Y=1)=\frac{P(Y=1)}{1-P(Y=1)}$, then the DR estimator for the marginal causal odds ratio is simply
$$\frac{\hat{\text{odds}}(Y^1=1)}{ \hat{\text{odds}}(Y^0=1)} = \frac{\frac{\hat{P}(Y^1=1)}{1-\hat{P}(Y^1=1)}}{\frac{\hat{P}(Y^0=1)}{1-\hat{P}(Y^0=1)}}$$
To get confidence interval you can bootstrap or use the delta method or M-estimation to estimate the marginal log odds ratio from the marginal probabilities.
-
$\begingroup$ If I inputted the inverted propensity score into a Logistic regression model along with covariates Xi and outcome Y, would I get the same results as doing this calculation manually @Noah? $\endgroup$– StatsBioCommented Oct 22, 2021 at 13:59
-
1$\begingroup$ No, because that is a different estimator. It is possible they are asymptotically equivalent, but this is the only way of computing this specific estimator. $\endgroup$– NoahCommented Oct 22, 2021 at 15:07
-