This is a question about backdoor criterion (as per J. Pearl) on finding causal effects. It is linked to a specific exercise in a specific book, but I hope it will be sufficiently generic and self-contained to be of general use.
Problem statement
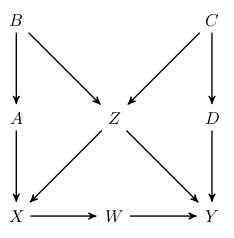
I am self-studying Pearl, Glymour, Jewell Causal Inference in Statistics, A Primer. Not quite sure about Q3.5.1 b. There we are given a causal diagram
And asked to find z-specific effect of X on Y, i.e.:
$$ P\left[Y=y\, \Big|\,do\left(X=x\right),\,Z=z\right] $$
As soon as we condition on $Z$, we are creating constraint that correlates $B$ and $C$ and thus opens a back-door $XABZCDY$. To estimate effect of $X$ on $Y$, that backdoor path needs to be broken. In my understanding, this can be done by conditioning on any of the $A$, $B$, $C$ or $D$.
Model solution
I also have model solutions (found online). There, only one option is mentioned - to condition on $C$:
$$ P\left[Y=y\, \Big|\,do\left(X=x\right),\,Z=z\right]=\sum_{c} P\left[Y=y\, \Big|\,X=x,\,Z=z,\,C=c\right]\cdot P\left[C=c\right] $$
Attempt to explain the model solution
Is there a reason why conditioning on $C$ is given as a sole solution? I can rule out conditioning on $A$ or $D$ since those are not independent variables. That leaves a question of whether I could condition on $B$.
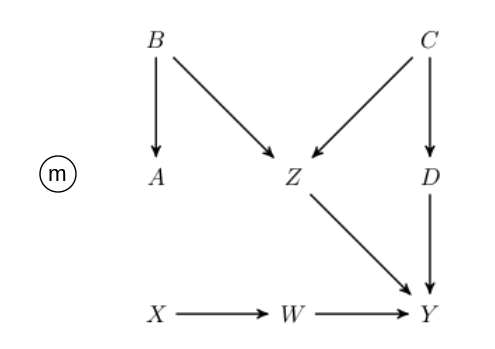
One way I can think of explaining why conditioning on $B$ would not work is by noting that causal effect corresponds to conditional probability on a modified diagram:
$$ P\left[Y=y\, \Big|\,do\left(X=x\right),\,Z=z\right]=P_m\left[Y=y\, \Big|\,X=x,\,Z=z\right] $$
Now, I can express this as:
$$ \begin{align} P_m\left[Y=y\, \Big|\,X=x,\,Z=z\right] &= \sum_{b} P_m\left[Y=y\, \Big|\,X=x,\,Z=z,\,B=b\right]\cdot P_m\left[B=b\right] \\ &=\sum_{c} P_m\left[Y=y\, \Big|\,X=x,\,Z=z,\,C=c\right]\cdot P_m\left[C=c\right] \end{align} $$
Since $B$ is independent, its probability would not be affected by modification of the diagram, so $P_m\left[B=b\right]=P\left[B=b\right]$, and same for $C$.
When it comes to conditional probability, we can use the fact that with fixed $Z=z$ there is no causal link from $C$ to $X$, thus conditioning on $C$ is the same on both the original and the modified diagram: $P_m\left[Y=y\, \Big|\,X=x,\,Z=z,\,C=c\right]=P\left[Y=y\, \Big|\,X=x,\,Z=z,\,C=c\right]$. This logic would not work for $B$ since $B$ does affect $X$ on the original diagram. Therefore we can only condition on $C$:
\begin{align} P\left[Y=y\, \Big|\,do\left(X=x\right),\,Z=z\right]&=P_m\left[Y=y\, \Big|\,X=x,\,Z=z\right] \\ &=\sum_{c} P_m\left[Y=y\, \Big|\,X=x,\,Z=z,\,C=c\right]\cdot P_m\left[C=c\right] \\ &=\sum_{c} P\left[Y=y\, \Big|\,X=x,\,Z=z,\,C=c\right]\cdot P\left[C=c\right] \end{align}
Does this make sense?