1
$\begingroup$

I run a standard GARCH (1,1) model and obtain the following results.

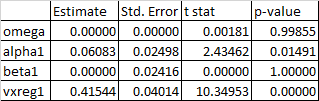
enter image description here

Thereafter, I add an external regressor in the same model and obtain the following results:

enter image description here

The GARCH coefficient (beta1) is zero and the p-value is 1. The coefficient of the external regressor (vxreg1) is 0.415 with p-value of 0.000.

I use the robust standard errors.

How to interpret the result? Should I concur that the external regressor reduces/removes volatility contemporaneously?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

Interesting. I would not say the external regressor reduces/removes volatility but it is able to fit/explain it better than the previous period's conditional variance $\sigma_{t-1}^2$ can. This reminds me a bit of the realized GARCH model. There, including lagged realized variance into the conditional variance equation tends to make the lagged squared return term not statistically significant; see slide 36 of Peter R. Hansen's "Lecture 3: Realized GARCH Models" (2016). Meanwhile, in your case the external regressor does the same for lagged conditional variance.

Improve this answer
$\endgroup$
8
  • $\begingroup$ Thank you very much for this explanation. Much appreciated. $\endgroup$
    – Jyoti Nair
    Commented Nov 2, 2022 at 8:33
  • $\begingroup$ @JyotiNair, you are welcome! I am still thinking why/how this would be happening. $\endgroup$
    – Richard Hardy
    Commented Nov 2, 2022 at 8:56
  • $\begingroup$ I ran a return equation of ARMA (6,3), as the optimised lags with minimum AIC score. If there is anyway I can share the data with you, and if you are keen, I will be more than happy to share. $\endgroup$
    – Jyoti Nair
    Commented Nov 3, 2022 at 7:27
  • $\begingroup$ @JyotiNair, thank you, but I am thinking more in theoretical terms here. $\endgroup$
    – Richard Hardy
    Commented Nov 3, 2022 at 10:55
  • 1
    $\begingroup$ @JyotiNair, well, you have a statistically significant $\alpha_1$, so past squared error has something to say about today's conditional variance, too, even when the external regressor is accounted for. $\endgroup$
    – Richard Hardy
    Commented Nov 7, 2022 at 10:52

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.