I am doing a statistical test (program used is SPSS). On the basis of distribution and sample size, I have to chose the correct variable analysis. I also have to justify every decision. I have two independent groups with a sample size of $n=43$ and $n=12$. My first step is to decide whether the data in groups is normally distributed.
As per instructions:
When deciding if data is normally distributed, I should take into consideration the use of a histogram or QQ plot along with a Kolgomorov-Smirnov or Shapiro-Wilk test. I should also consider mean and median difference, skewness, and kurtosis values .
If $n>30$, I should consider data being normally distributed if the deviation is not greater than moderate.
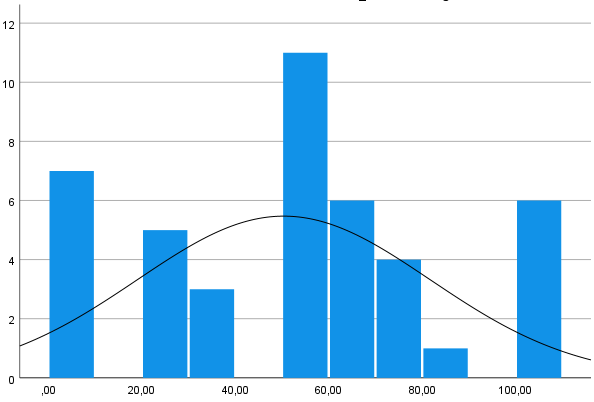
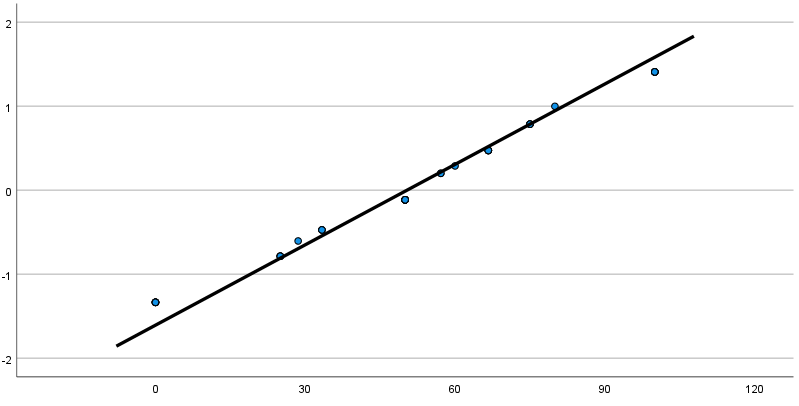
Now regarding Group 1, there exists a non-existent mean/median difference and kurtosis/standard error and skewness/standard error point to normality, but Kolgomorov-Smirnov and Shapiro-Wilk test values are less than $0.05$. The QQ plot shows values gathered in a line. The data in the histogram seems to have close to symmetric distribution, but how do extreme values at 0% and 100% affect my decision if data is normally distributed? Is the data normally distributed?
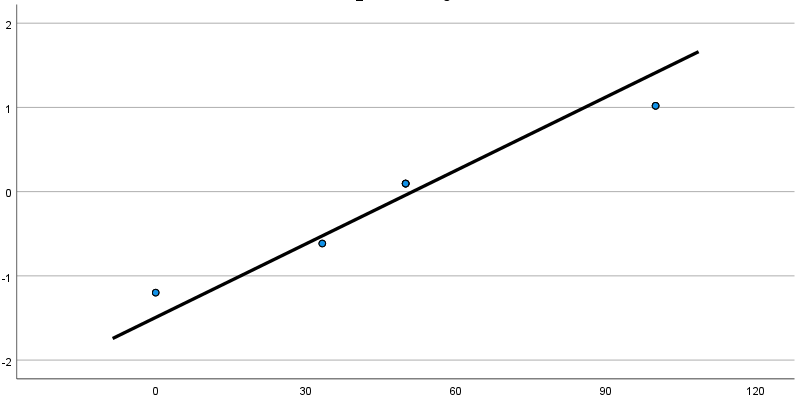
Regarding Group 2, I am pretty much lost. Mean/median difference, kurtosis/standard error, skewness/standard error and the SW-test does not seem point to deviation, but the KS test has a value of 0.019. In the histogram, there is also an extreme value at 100% that I don't know how to relate to. How does it affect normality? A small sample size of $12$ ($n<30$) doesn't allow one to consider a normal distribution (if the deviation is not greater than moderate). Does this data have a normal distribution?
When considering the normality of a distribution, is there a "grade" regarding histograms, QQ plots, skewness/kurtosis, Kolgomorov-Smirnov and Shapiro-Wilk tests, etc.?
If one group has a normal distribution but the other does not, and considering small sample in Group 2, should I continue with a non-parametric test? Also, how do I decide if the deviation is moderate?
Group 1 Descriptives:
- Mean=50.4
- Median=50
- Standard Deviation = 31
- Kolgomorov-Smirnov = 0.021
- Shapiro-Wilk = 0.012
- Kurtosis/standard error = -0,162
- Skewness/standard error = -0,024
Group 1 Histogram
Group 1 QQ plot
Group 2 Histogram
- Mean = 51.5
- Median = 50
- Standard Deviation = 34.4
- Kolgomorov-Smirnov = 0.019
- Shapiro-Wilk = 0.060
- Kurtosis/standard error = -0,057
- Skewness/standard error = 0,017
Group 2 QQ plot
Part 2 (In reference to posted answer)
Regarding the equality of variance assumption. I assumed equality of variance is examined by doing Levene's test for equality of variances. I proceeded to test the data for equality of variance and got the following results. The sig. value was 0.955. That's pretty good value, right? I suppose that the assumption of homogeneity of variance has been met?
Now regarding the sample sizes of my groups not being equal. It was some time ago and I can't find direct quote but basically, the author said that the larger the difference between sizes of sample groups, the larger the Sig. of Levene's test should be in order to use Independent T-test. Is this correct? If so, is sig. value of 0.955 enough?
You also noted the gaps between bars in the histogram. I was wondering the same thing. I went through all variable values and found that some values (that were very close) in the histogram for group 1 have been lumped together, although not for group 2. I asked a teacher about this but he said that histograms looked ok and I should use them as they are. Now I should note that the initial sample size for the whole variable was 1000 but I had to filter it for different parameters.
If assumptions are met, I would like to stick to the independent t-test as a first choice because we haven't discussed Welch's in this course. Even the course literature refers to "t-test with corrected degrees of freedom" not being discussed. Now I'm translating directly but I assume it's referring to Welch's or something similar. I believe as long as my line of reasoning is logical and I account for weaknesses when I'm justifying my choice, I think I'm good. Feel free to let me know if my interpretations are wrong in any way.