1
$\begingroup$

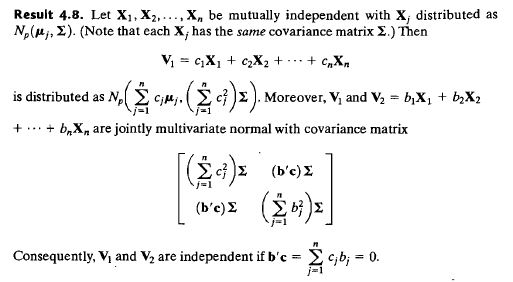
Let $X_1,...,X_4$ be independent $N_p(μ,Σ)$ random vectors. Let $V_1,V_2$ be such that $$V_1=(1/4)X_1-(1/4)X_2+(1/4)X_3-(1/4)X_4 $$ $$V_2=(1/4)X_1+(1/4)X_2-(1/4)X_3-(1/4)X_4 $$

I need to find the marginal distributions of $V_1$ and $V_2$ and the joint density. Since they are linear combinations of random vectors I do not know the theory behind it to solve this. Any answers will be much appreciated. Thanks

Improve this question
$\endgroup$
4
  • $\begingroup$ This looks like routine book-work - please read the link and modify your question and tags as needed. You should at least know the basic results about expectations, variances and covariances, and I presume you know that linear combinations of multivariate normals are multivariate normal. That should actually be sufficient here (though not automatically the simplest way to approach it). $\endgroup$
    – Glen_b
    Commented May 13, 2014 at 0:11
  • $\begingroup$ @Glen_b I should have mention that I only require how to compute the mean and co-variance I already knew it should be normal. $\endgroup$
    – Heisenberg
    Commented May 14, 2014 at 6:27
  • $\begingroup$ en.wikipedia.org/wiki/Expected_value#Linearity and en.wikipedia.org/wiki/Variance#Basic_properties $\endgroup$
    – Glen_b
    Commented May 14, 2014 at 6:39
  • $\begingroup$ @Glen_b thanks. I added a part from a text book in the answer below which also addresses the issue $\endgroup$
    – Heisenberg
    Commented May 14, 2014 at 6:42

2 Answers 2

Reset to default
3
$\begingroup$

Use the following notation: Let $X$ be the 4 by $p$ matrix of your $X$ variables. Let $M$ be a 2 by 4 matrix of coefficients. Then $V=MX$ is your new variable. The distribution of $V$ is multivariate normal, with variance $M \Sigma M'$. To get the mean, apply the $M$ transformation to the means of the $X$ variable. Looks as if it should be 0.

Mardia's book on multivariate analysis is a good reference for this sort of problem.

Improve this answer
$\endgroup$
5
  • $\begingroup$ So what you are suggesting is to solve this the same way as say X is $N_p(μ,Σ)$ any linear combination of its elements are also normally distributed? Since this is a set of X's with $N_p(μ,Σ)$ what is the theory behind your answer? If it was the case for a linear combination of elements of one X then using the properties of multivariate normal distribution it can be solved. You have done the same if I am not wrong but how is that possible for random vectors? $\endgroup$
    – Heisenberg
    Commented May 12, 2014 at 14:06
  • 1
    $\begingroup$ The theory is that linear combinations of normals are normal. That's basically what you are doing here. $\endgroup$
    – Placidia
    Commented May 12, 2014 at 14:17
  • $\begingroup$ I have posted below a result that I found. Do you think this is the same as your answer? $\endgroup$
    – Heisenberg
    Commented May 13, 2014 at 14:58
  • $\begingroup$ is this the same? $\endgroup$
    – Heisenberg
    Commented May 14, 2014 at 6:26
  • $\begingroup$ Yes, that answers the question. Nice. $\endgroup$
    – Placidia
    Commented May 14, 2014 at 13:51
3
$\begingroup$

enter image description here

I found this result from Johnson And Wichern's book. Adding this to the answers hoping that it would benefit someone

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.