2
$\begingroup$

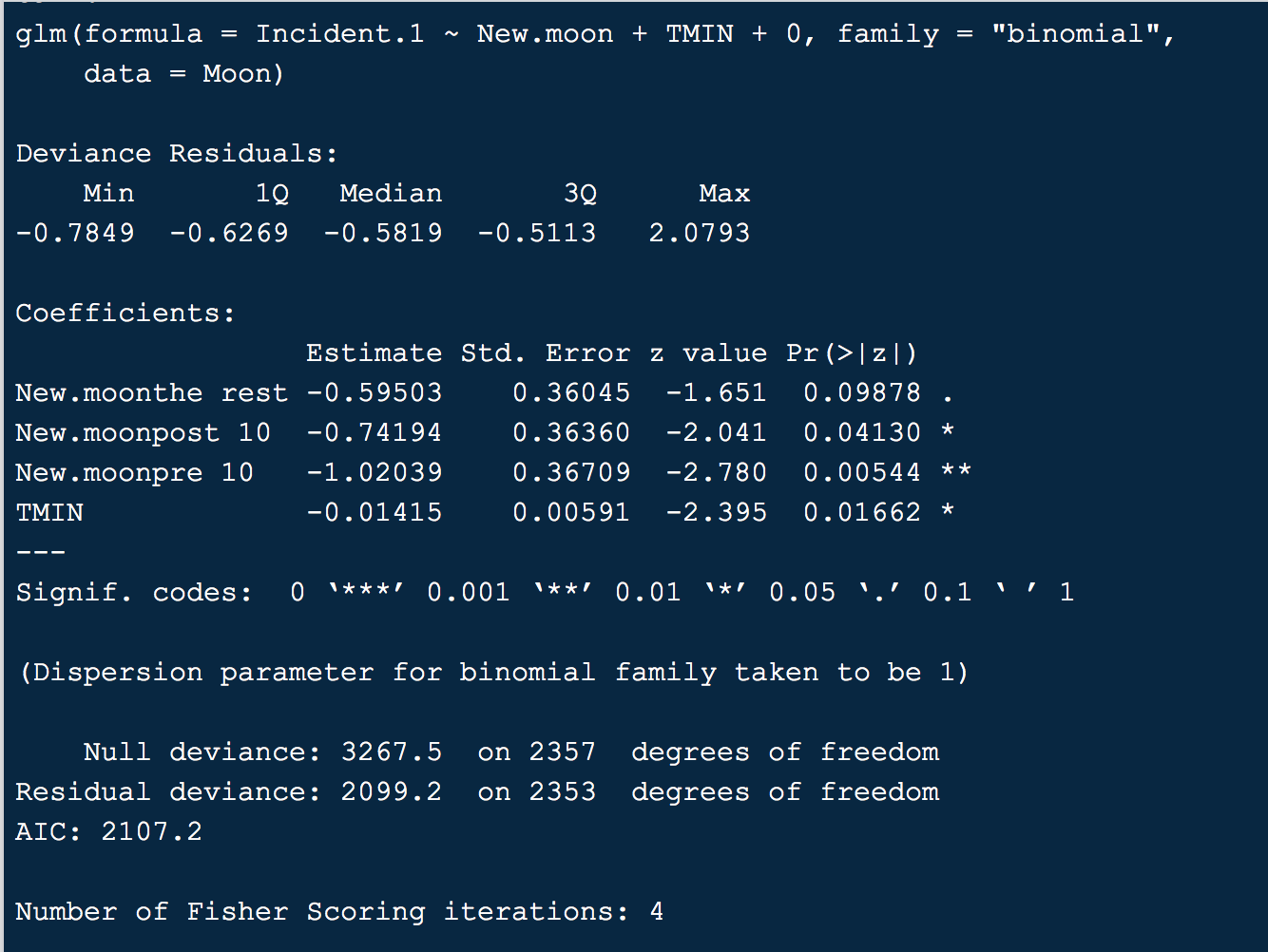
So I ran a binomial glm to look at the effect of minimum temperature (continuous data) and moon phase (categorical data with 3 categories) on lion incidents. I removed the intercept to look at all 3 categories of moon by using + 0 in my glm. These were the results:

Glm code and result

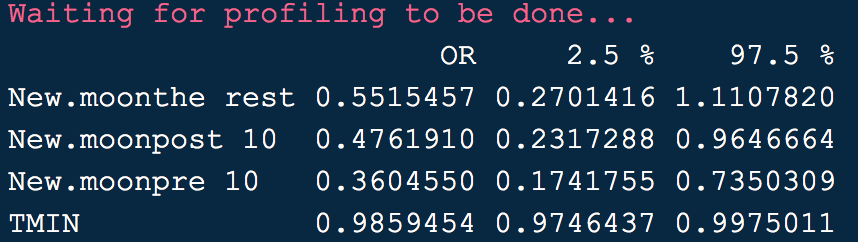
I then calculated the odd ratios and confidence intervals using the code:

exp(cbind(OR = coef(GLMoon), confint(GLMoon)))

which gave me this:

enter image description here

I'm not fully clear on how to interpret these as most of the odds ratios I've seen have been above 1, but from what I've read I guess it would mean that the odds for incidents during the 'new.moonthe rest' phase are 45% lower than during other phases? And that holding moon phases at a fixed value, we will see a 1.14% increase in the odds of an incident, for a one-unit decrease in temperature?

I'd prefer to calculate probabilities of attack, but I'm unsure how to do this from the coefficients or odds ratios when the intercept is removed. I'd really appreciate any help!

Cheers

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

For the various levels of New.moon, these are not odds ratios, but odds. So the odds of an "incident" is $0.55$ during the 'new.moonthe rest' phase when TMIN (minimun temperature) is at 0. You could also back-translate this into the chances of an incident (i.e., $0.55 / (1 + 0.55) \approx 0.35$).

If you want an odds ratio, you have to compare two odds against each other. So, for example, the odds are $0.55$ for the 'the rest' phase and $0.36$ for the 'pre' phase. So, the odds ratio is $0.55 / 0.36 \approx 1.53$, or in other words, the odds are $1.53$ times higher during 'the rest' phase compared to the 'pre' phase.

For TMIN, the value is an odds ratio, comparing the odds of an incident for a one-unit increase in minimum temperature (so the odds ratio of $x+1$ versus $x$, where $x$ is the minimum temperature value).

Improve this answer
$\endgroup$
6
  • $\begingroup$ Thanks Wolfgang, really helpful. So back-translating into a chance of an incident means there's a 35% chance of an incident during the 'the rest' phase when temperature is at 0? None of my minimum temperatures went to 0 though, so how do I relate this to the actual temperature values? I assumed it was a 35% chance of attack when temperature values remained the same? Was my interpretation of the TMIN odds ratio then kind of correct? 1.14% increase in the odds of an incident, for a one-unit increase (I said decrease) in temperature? How do I convert my TMIN odds ratio value into a probability? $\endgroup$
    – Josh Robertson
    Commented Aug 21, 2016 at 18:54
  • $\begingroup$ Yes, the 35% applies when temperature is at 0. But then it is an extrapolation and you probably shouldn't interpret it. Of course you can easily estimate the odds (and hence chances) for other temperature values based on the model. For TMIN, the odds ratio is .986, so as temperature increases, the odds decrease (and vice-versa). If you want to phrase it in terms of a one-unit decrease in temperature, you should say that the odds increase by $(1-.986)*100 = 1.4$%. $\endgroup$
    – Wolfgang
    Commented Aug 21, 2016 at 19:15
  • $\begingroup$ Hi @Wolfgang - I'm revisiting this problem because I've been told I can't remove the intercept from my GLM. Given this, my OR results are now: Intercept (Which is my moonphase 1) = 0.303, moon phase 2 = 0.757 , and moonphase 3 = 1.158. I'm having real difficulty interpreting these to find the odds for each phase, and subsequently comparing the odds of an incident from phases 1 and 3 to phase 2. You mentioned previously that my output showed me odds during each incident rather than odds ratios - have they been added onto each other for this? I'd really appreciate some help on this. Thanks! $\endgroup$
    – Josh Robertson
    Commented Jul 26, 2017 at 12:10
  • 1
    $\begingroup$ @JoshRobertson Here is an example that walks you through a logistic regression model and its interpretation: stats.idre.ucla.edu/r/dae/logit-regression $\endgroup$
    – Wolfgang
    Commented Jul 26, 2017 at 13:02
  • $\begingroup$ Perfect, thanks very much @wolfgang I'll go through it now. $\endgroup$
    – Josh Robertson
    Commented Jul 26, 2017 at 13:05

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.