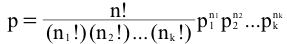Probability of each of the three Christmas puddings having exactly 2 coins

Question

A cook makes plum puddings for Christmas. He stirs 6 coins into the pudding mixture thoroughly before dividing it into three equal portions. What is the probability there are 2 coins in each pudding?

The answer is 10/81, but I cannot work out how to arrive at this answer.

---

What I worked out so far:

A = pudding A has exactly 2 coins

$P(A) = P(B) = P(C) = {6\choose 2}(1/3)^2(2/3)^4$

$P(A~\text{or}~B~\text{or}~C) = 3P(A) - 2P(A~\text{and}~B~\text{and}~C)$ [because $P(A~\text{and}~B) = P(A~\text{and}~B~\text{and}~C)$]

so

$P(A~\text{and}~B~\text{and}~C) = 3P(A)/2P(A~\text{or}~B~\text{or}~C)$

so I guess I just need to figure out what $P(A~\text{or}~B~\text{or}~C)$ is.

Answer 1

Call the number of pieces in each section $A$, $B$, and $C$. Because $A+B+C=6$, you are interested in $Pr(A=2, B=2) = Pr(B=2|A=2)Pr(A=2)$.

$Pr(A=2)$ is a simple binomial calculation: $A\sim Binom(6, 1/3)$, so $Pr(A=2) = {6\choose 2}(1/3)^2(2/3)^4 = 80/243$.

Conditioned on $A$ having two pieces, $B\sim Binom(4, 1/2)$, so $Pr(B=2|A=2) = {4\choose 2}(1/2)^4 = 3/8$.

Multiplying these together, we conclude that $Pr(A=2, B=2) = 10/81$.

Answer 2

You shouldn't use the binomial distribution here as it is a multinominal distribution problem (a generalization of the binomial).

So let's gather what we have:

n = 6 (total number of events)
n1 = 2 in part 1 (pudding #1)
n2 = 2 in part 2 (pudding #2)
n3 = 2 in part 3 (pudding #3)
p1 = 2/6 (probability to get 2 from n1)
p2 = 2/6 (probability to get 2 from n2)
p3 = 2/6 (probability to get 2 from n3)

the formula goes like this:

so let's put the numbers in motion:

$p = \frac{6!}{(2!*2!*2!)} *(\frac{2}{6})^2 * (\frac{2}{6})^2 * (\frac{2}{6})^2 = 0.12345 $

and we get

10/81