Difference in Difference - Does the control units need to be similiar?

Question

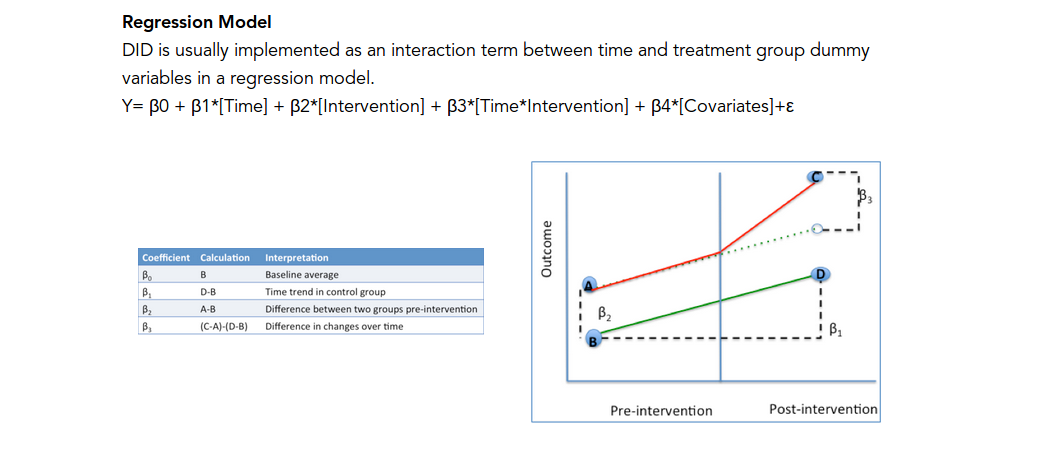
This is often shown as the setup and simple analysis for a difference-in-difference estimation. Lets say that the control series is significantly smaller than the treated series. Below this means the green line would be greatly shifted downward - but still similar trend as red (the primary assumption of d-i-d). Does the (C-A)-(D-B) still work if the outcome value for the series is not close to each other? It seems like we would need to use percentage change.

Here is an example. The d-i-d estimate is 963. It doesn't make sense though to me to adjust the increase in the treatment market by the change in the control when the sizes are so different. It seems like a better approach would be to look at the growth in control (20% here) and apply that to the treated: 1.2*1530 and then compare this against the actual of 2500. In this case the estimate would be 664.

It seems like we should compare the treated market growth to the % change in the control market because as is, we remove from the treated increase the absolute value of the control and that seems wrong, since the starting base for the control is so much lower. 7 units is 20% increase for the control, but is very small compared to the treated.

Answer 1

I think the problem is that the "parallel trends" (or parallel paths) assumption here was confused with a "parallel growth" assumption. Two lines are parallel over time when the distance between the two lines remains the same at each point in time. But this is not true when you compute 1.2*1530 (you can easily visualize this).
Instead, the control group increases their outcome by 7 in the second period by going from 35 to 42. The outcome of the treated moves in a parallel fashion only if they also increase their outcome by 7. So the point at the end of the dashed line in your graph for the treated, i.e. the unobserved counterfactual outcome for the treated in the absence of the treatment, should have value 1537. Going from this counterfactual point to the observed point C then yields the solution, 2500 - 1537 = 963.

You raise two very good points though with this question:

1. Even though we care about parallel trends in a diff-in-diff setting and not so much about the initial difference between the treatment and control groups, this assumption is sensitive to functional form. Whilst the outcome series 35-42 and 1530-1537 are parallel in levels they will NOT be parallel in logs. This leaves scope for people to fish for results. Don't like your diff-in-diff graph? Try logs!
2. In the very basic specification above the following is not much of a problem. But if you have multiple periods and you want to control for time trends by including dummies and a parametric linear time trend which is specific to the treatment and control groups, this changes the underlying assumptions of the diff-in-diff estimator. See this World Bank post by Jed Friedman and the corresponding paper he refers to. Admittedly this point is a little off-topic but was fitting with the "parallel trends" vs "parallel growth" issue.