3
$\begingroup$

I'm trying to understand (esp. the boldfaced part) how THIS ARTICLE (see p. 174, top-right corner) is suggesting that:

"to use the confidence intervals to test a statistical hypothesis and to maintain a Type I error rate at alpha:

  1. When testing a two-sided hypothesis at the alpha level, use a 100(1 - alpha)% confidence interval.

2. When testing a one-sided hypothesis at the alpha level, USE a 100(1 - alpha / 2)% confidence interval."

Improve this question
$\endgroup$
2
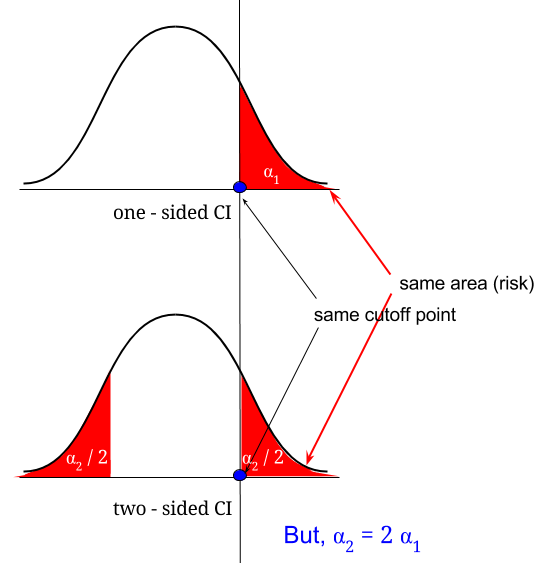
  • $\begingroup$ The article has 100(1 - 2 alpha) as Antoni writes in his answer. But you confusingly have (incorrectly) alpha/2 in your question. $\endgroup$
    – PatrickT
    Apr 1, 2018 at 21:13
  • $\begingroup$ A citation for the article which the OP mentions: Steiger JH. Beyond the F test: Effect size confidence intervals and tests of close fit in the analysis of variance and contrast analysis. Psychol Methods. 2004 Jun;9(2):164-82. doi: 10.1037/1082-989X.9.2.164. PMID: 15137887. $\endgroup$
    – dipetkov
    Oct 6, 2022 at 9:35

1 Answer 1

Reset to default
2
$\begingroup$

If you generate the two-sided confidence interval with a confidence level of $95\%$ (or $\alpha_1 = 5\%$), the cut-off points (or endpoints) of the interval will leave out a probability of a type I error of $\frac{1}{2} \alpha=2.5\%$ on either end.

If you are performing a one-sided test, and want to preserve a risk $\alpha = 5\%$ of rejecting the null when it is in fact true, you will want to generate a two-sided CI with and confidence level of $90\%$ to leave $5\%$ probability at each end.

So you double the initial $\alpha_1 =5\%$ to $\alpha_2=2\alpha_1=10\%.$

Hence the quote:

  1. When testing a two-sided hypothesis at the alpha level, use a $100(1 - \alpha )\%$ confidence interval.
  2. When testing a one-sided hypothesis at the alpha level, use a $100(1 - 2 \alpha)\%$ confidence interval.

enter image description here

Improve this answer
$\endgroup$
15
  • $\begingroup$ Antoni, I'm a bit confused. Isn't it true that when we do a one-sided test, we have one-rejection region? If yes, where do we place our rejection region under the sampling distribution? $\endgroup$
    – rnorouzian
    Jan 24, 2017 at 18:56
  • $\begingroup$ Yes. It is true. Just imagine the area under that rejection level in red. It contains, say $5\%$, which is the probability of rejecting the null when it is true. Now picture the other end of the "bell curve" without any areas in red. $\endgroup$
    – Antoni Parellada
    Jan 24, 2017 at 18:58
  • $\begingroup$ If you want to get that same area (with its cutoff point on the $x$-axis by "cheating" and actually getting two red areas... $\endgroup$
    – Antoni Parellada
    Jan 24, 2017 at 18:59
  • $\begingroup$ one at either end of the curve (a $\text{mean}\pm \text{1/2 CI}$)... $\endgroup$
    – Antoni Parellada
    Jan 24, 2017 at 19:00
  • $\begingroup$ ... still preserving the original $5\%$ red area that you had at the beginning, you will have to double $\alpha,$ $\endgroup$
    – Antoni Parellada
    Jan 24, 2017 at 19:01

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge that you have read and understand our privacy policy and code of conduct.

Not the answer you're looking for? Browse other questions tagged or ask your own question.