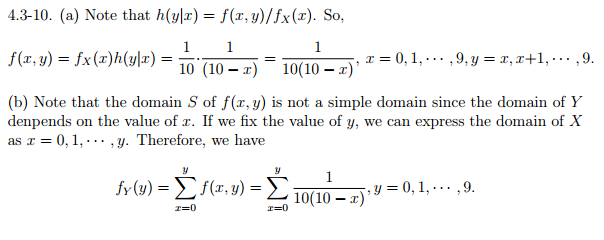I have the solution to this problem but I don't really understand it.
Let $f_X(x) = \dfrac {1}{10}, \ x = 0, 1, 2, ... 9$ and $h(Y|x) = \dfrac {1}{10-x}, y = x, x+1, x+2, ..., 9$.
a) Find $f(x, y)$.
b) Find $f_Y(y)$
I am having trouble understanding part b). As I recall, $\displaystyle f_Y(y) = \sum_{x \in S_x}f(x, y)$. I see that $S_Y$ is not fixed, but $S_X$ is. So why did my professor write $f_Y(y) = \displaystyle \sum_{x=0}^y \dfrac {1}{10(10-x)}$ instead of $f_Y(y) = \displaystyle \sum_{x=0}^{9} \dfrac {1}{10(10-x)}?$ The first one seems to imply that the domain of $x$ is not fixed.
EDIT: As I was writing this question, I think I may have figured it out, but I would still like confirmation please that I am correct:
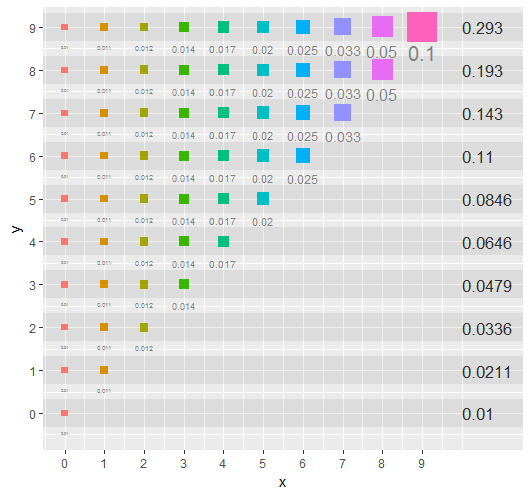
Take $y = 3$ as an example.
$\displaystyle f_Y(y) = \sum_{x \in S_x}f(x, y) = f(0, 3) + f(1, 3) + f(2, 3) + f(3, 3) + f(4, 3) + f(5, 3) + f(6, 3) + f(7, 3) + f(8, 3) + f(9, 3) = \sum_{x=1}^3 \dfrac {1}{10(10-x)} + 0 + 0 + 0 + 0 + 0+0$