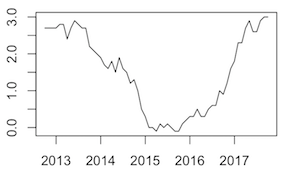
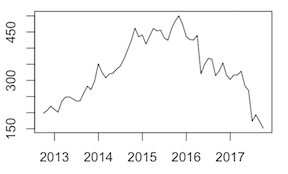
I wish to test the cross-correlation of two time series to measure how "instantaneously related" my time series are. However, I am not quite sure if I should difference my time series first, and make them stationary? Because they obviously aren't:
And the cross-correlation I get is very different if I difference my time series, or not.
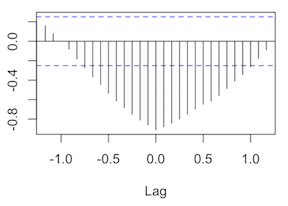
Cross-correlation cff() on original non-differenced data:
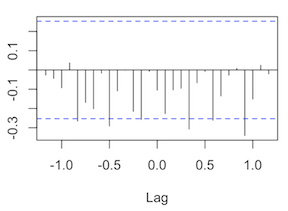
Cross-correlation cff() on differenced data with diff():
Which one is right? Am I supposed to use cff() on stationary data only? Thank you for the help.