1
$\begingroup$

I took two separate water samples (one sample per bottle) in twenty one river locations (n=42). For the purpose of this question I am calling the two bottles duplicates.

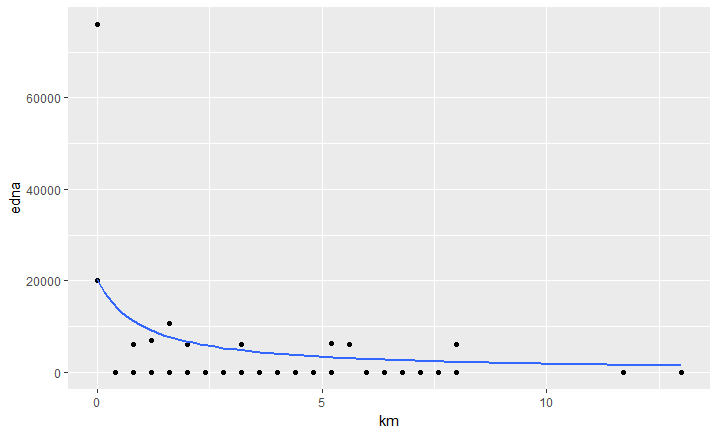
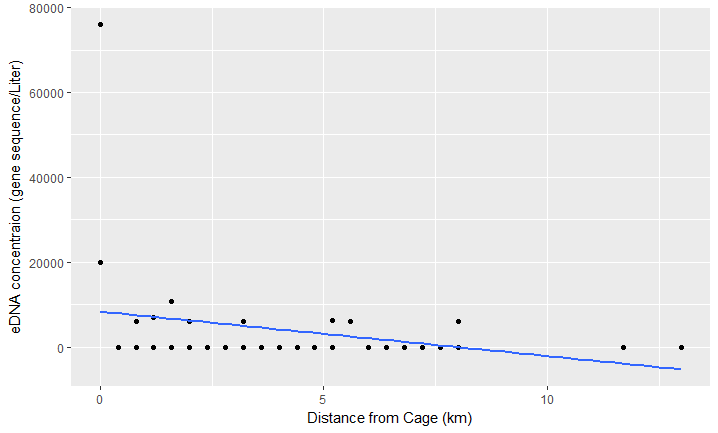
I am trying to show how environmental DNA (eDNA) attenuates with river distance from a cage of organisms. So the samples were taken every 0.4 km up to 13 km from the caged organisms. However, in many locations one sample tested positive for eDNA and the other was a non-detect.

For the purpose of plotting the data I assigned the non-detects a 0. Is this correct? I tried running a linear regression and non-linear regression (See plots below).

enter image description here

I don't think this is a good option since only 11 of my samples tested positive for eDNA and I do not know if it was correct to assign a 0 to the non-detects. However, I am providing the plots so you can see what my data looks like. Please note there are two samples for every km interval (if there looks like there is only one it is because the values are the same).

Someone also suggested a Gaussian GLM, but I am not sure if that is correct either? And if so, how do I deal with the non-detects?

I am looking for some type of statistical analysis to show how eDNA attenuates with distance. Any suggestions with my data set? Would running a Monte Carlo analysis here be a good option?

enter image description here

Improve this question
$\endgroup$
3
  • $\begingroup$ It is very important to know the reason why two samples from the same location can have such different values. Is it the inaccuracy of the testing method or the sampling variance? This makes a world of difference. $\endgroup$
    – Jim
    Commented Feb 15, 2018 at 13:16
  • $\begingroup$ It is the sampling variation-the testing method is good. This is just a common issue with eDNA. $\endgroup$
    – Ellen
    Commented Feb 19, 2018 at 22:50
  • $\begingroup$ 1) Is this a common issue? If so, how is this dealt with in others studies? 2) From your plots it is clear that non-linear regression is much preferred over linear regression (could you provide your code perhaps). 3) A very crude option you could consider is averaging each of the "duplicates" and regressing those averages on distance. $\endgroup$
    – Jim
    Commented Feb 20, 2018 at 12:30

1 Answer 1

Reset to default
0
$\begingroup$

Don't have the rep to comment otherwise I wouldn't post such a vague answer. This isn't my area of expertise but ecologists have treated distance data like this with a set of related methods called Distance Sampling. I would suggest looking into this. You can basically bin your data so you get a histogram, then fit a 'detection function' curve to this.

Those applications are slightly different to what you are trying to do as they assume a uniform distribution is the reality, and detection decays away from the observer (the cage in your case), and they use the methods to estimate abundance of an organism. But there is no reason you can't use the software to fit the non-linear curves for your use-case. There is bespoke windows software or also R packages.

If I recall correctly there are a bunch of choices for the function, half-normal and hazard rate function are both there.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.