I'm analyzing a randomized controlled trial. At baseline time, the outcome variable of the control group (C) was slightly higher than that of the intervention group (I), but such difference wasn't significant.
At the end of the trial (3-month time), outcomes both groups had higher values than the baseline. Within-group change among (C) was not significant but within-group change among (I) was significant. Between-group change wasn't significant.
One member of the team thought that shouldn't be the case, i.e., if (I) showed significant within-group change, that would lead to significant between-group difference.
I think I need to convince with some formal proof. That is to show that, on theory, that can happen. Can you tell some relatively simple mathematical reference for this sort of test? "Light" math is preferable though.
EDIT to take into account Frans Rodenburg's comment.

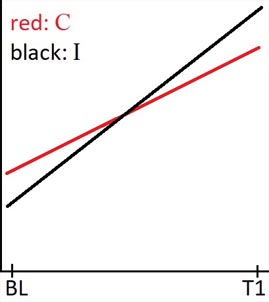
(C)and(I)is insignificant after 3 months, but I'll assume it was. This can happen for example if the control group has higher variance than the intervention group, rendering the comparison between them insignificant, while the before/after difference is significant for the intervention group. Is the difference still significant after correcting for 4 hypothesis tests? $\endgroup$