I hope someone would be able to shed some light as to why equation 1.33 is the way it is from first principles. It looks a lot like the case where the initial state is randomised but the final state to which the Markov chain is, is fixed. From the expression, there are two probability terms which isn't clear to me why this is so.
Any help is appreciated.
(1.33) is just the Law of Total Probabilities:
$$ P(A) = \sum_k \left[ P(B_k) P(A | B_k) \right], $$
where $\{B_k \;|\; k = 0, \ldots\}$ is a countable partition of the sample space.
In this case, clearly having $k$ children (in the first generation) is a countable partition of the sample space, and $P(B_k) = p_k$ is the probability for this.
Suppose there are $k$ children in the first generation. Then, under this model, they all peter out or not independently. Consequently, $P(A | B_k) = \rho^k$ is the probability of petering out assuming $k$ children in the first generation.
By the Law of Total Probabilities, the sum of this product is the overall probability of extinction.
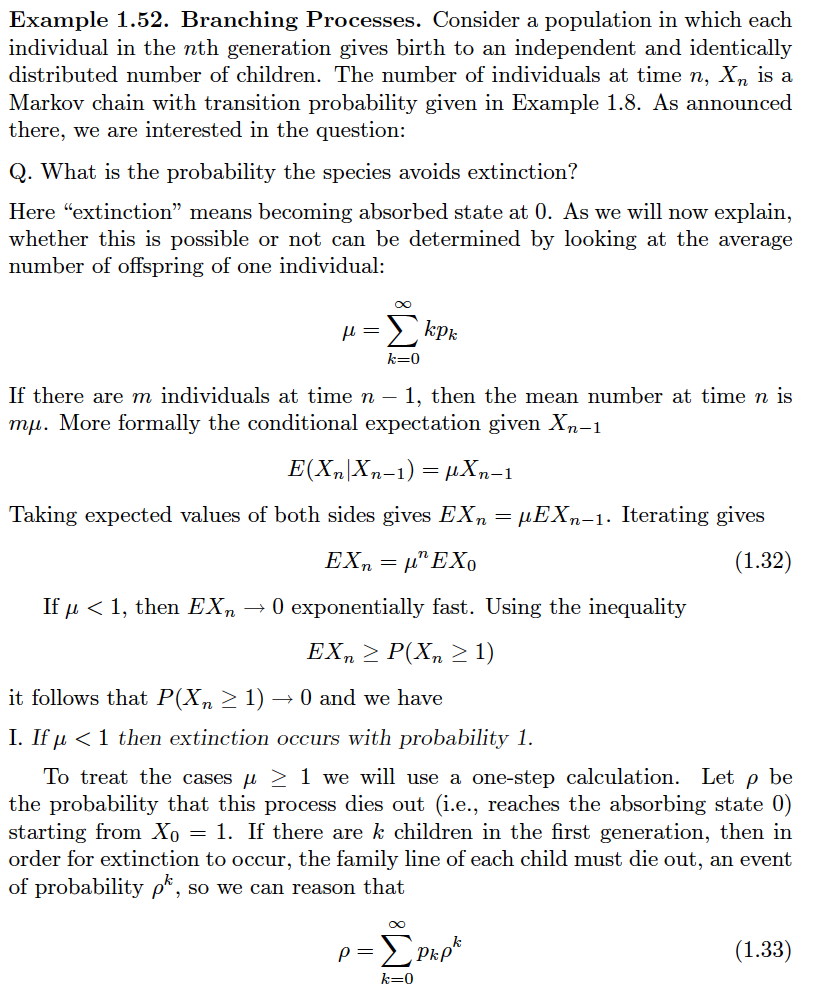
The reason this argument works is that, in a pure branching process, lineages don't interact in any way after they've branched off each other. It's as if every individual was alone in the world, never even seeing any others of its kind (except for its own parent, which it saw once when it was born, and its own offspring that also disappear somewhere far away immediately after being born).
What this means is that, if we initially have several (presumably widely separated) individuals living in the world, each of those individuals will be the founder of an independent lineage, and each of those lineages will either survive or die off independently of the others. Thus, if we know that a single lineage dies off with probability $\rho$, then the probability that $k$ separate lineages all die off independently of each other is simply $\underbrace{\rho \times \rho \times \dots \times \rho}_{k \text{ times}} = \rho^k$.
But if we know that the probability of a single individual producing $k$ offspring is $p_k$, then that means that the probability of the individual producing $k$ offspring and all the lineages of those offspring then dying off is $p_k \times \rho^k$. And we can add up these probabilities for all possible offspring counts $k$ to obtain the total probability $\sum_{k=0}^\infty p_k \times \rho^k$ of all the lineages of a single individual's offspring eventually dying off.
But the lineage of an individual dies off if and only if the lineages of all its offspring die off, so this total probability must be equal to just $\rho$! And thus we get the "equation 1.33" that you cite: $$\rho = \sum_{k=0}^\infty p_k \, \rho^k$$
