One Way ANOVA, 3 groups with unequal sample sizes [closed]

Question

I have a question regarding one way ANOVA in order to see whether there is a significant difference using SPSS; I have 3 groups of unequal sample sizes (n=7, n=7 and n= 13). I know that the sample sizes are small, and I can't therefore find the suitable post-hoc test for this analysis of data. I tried the Tukey test, but it was not working...?

Answer 1

The problem you are describing stems from having entered your data in parallel format (each group is recorded in its own column) vs. in serial format (each group is recorded in the same column, but there is a second column indicating the group membership).

Using your data, it should appear in SPSS in two columns like this:

1   18.67
1   39.88
1   19.33
1   3.17
1   5.77
1   13
1   10.18
2   10.86
2   9.5
2   5
2   13
2   3.9
2   7.94
2   7.38
2   16.82
2   11.67
2   13.31
2   9
2   12.79
2   14.1
3   14.6
3   22.9
3   16.38
3   6.77
3   2.75
3   13
3   25.5

With data in this format, you will be able to run the ANOVA and select the post hoc follow-up options (and they will run).

Hope this helps.

Answer 2

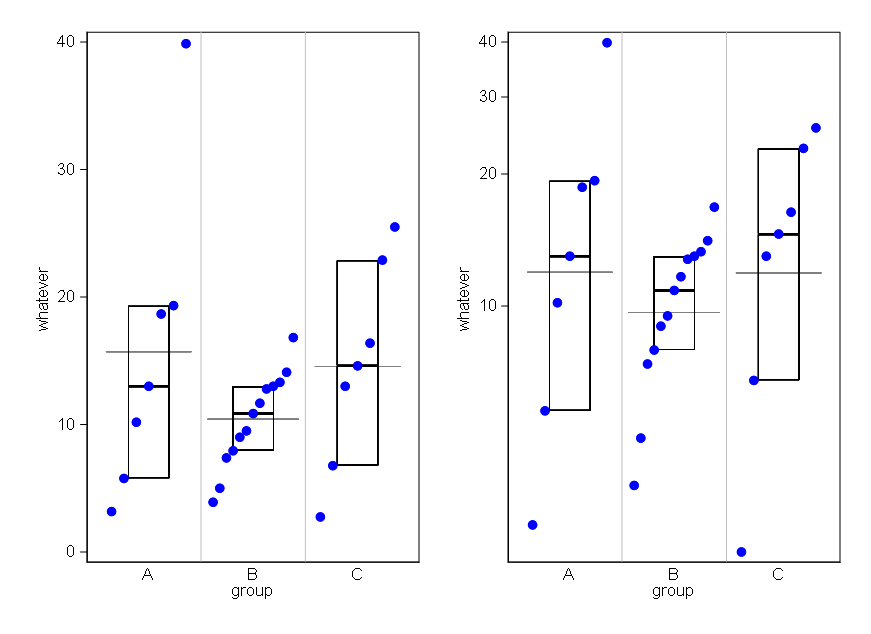
There are always plenty of votes for post hoc, so here is one for ante hoc, looking at the data in exploratory manner to see what they show and how that might guide the analysis. (The same Tukey really is the name to invoke too.)

I suggest that with plain ANOVA most conclusions will hinge on the outlier at 39.88, which pulls up the mean for A mightily.

On the face of it, working on logarithmic scale would not just pull in that outlier but might make more sense.

The display on the left is just a couple of twists away from a fairly conventional box plot, the twists being

1. Display of all points as a superimposed quantile plot. (A more prosaic vertically-aligned dot plot would work almost as well, but not quite.) In Parzen's terms, that is a quantile-box plot, although (side issue) that term appears with other meanings in statistical literature. If you want to follow that path, see any or all of

How to visualize independent two sample t-test?

How to use boxplots to find the point where values are more likely to come from different conditions?

How do I get which experiment is doing better using the Mann-Whitney U Test?

How can I measure difference between non-parametric data with many zeros?

2. Horizontal reference lines show the means.

The display on the right is the same idea, except plots are on logarithmic scale and the reference lines are geometric means.

Pessimists may want to shout ad hoc, but that just stands for (even if the translation is new to you) "fit for purpose". I note that the ordering A C B is the same for means and geometric means, but not for medians.

If I were advising for real, I would first ask what is being measured as a response variable and try to work out whether logarithmic scale made scientific or practical sense for that response.