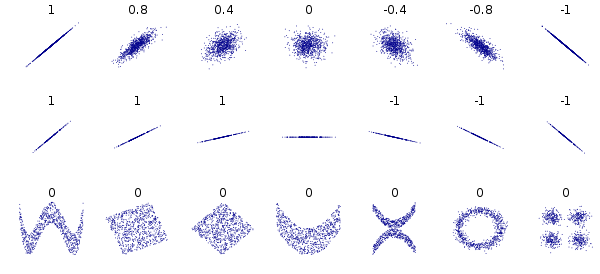
Kendall's tau measures the concordance between the two observations. For example,
for $(X_1, Y_1)$, and $(X_2, Y_2)$,
the pair is said to be concordant if
$X_1 > X_2$ and $Y_1 > Y_2$, or
$X_1 < X_2$ and $Y_1 < Y_2$,
otherwise, they are discordant. Hene, Kendall's tau is calculated as the difference between the probability of concordance and the probability of discordance.
I read many articles about the consistency of Kendall's tau. I find that Kendall's tau is not consistent. That is, Kendall's tau may indicate that the two variables are independent while they are not. I really do not understand that because since Kendall's tau give us the average of the concordance and discordance, then It should be consistent.
Here is one example of what I have read:
From the abstract of Bergsma and Dassion, 2014[1]:
"The most popular ways to test for independence of two ordinal random variables are by means of Kendall’s tau and Spearman’s rho. However, such tests are not consistent, only having power for alternatives with “monotonic” association."
and from the introduction of the same source:
"A drawback for certain applications is that τ and ρS may be zero even if there is an association between X and Y, so tests based on them are inconsistent for the alternative of a general association."
Here the authors said that Kendall's tau and Spearman's rho may be zero even if there is an association! I did not really understand this point.
My question is, why is Kendall's tau not consistent?
Help with an example would be much more appreciated.
[1] Bergsma, W. and Dassios, A. (2014),
"A consistent test of independence based on a sign covariance related to Kendall’s tau"
Bernoulli 20(2), 1006–1028
https://projecteuclid.org/download/pdfview_1/euclid.bj/1393594014
(also at https://arxiv.org/pdf/1007.4259.pdf)