A question about Trivariate Normal Distribution

Question

Question:

Let $(X_1,X_2,X_3)\sim N_3\left[\mathbf0, \begin{pmatrix}1&\rho_{12}&\rho_{13}\\\rho_{12}&1&\rho_{23}\\\rho_{13}&\rho_{23}&1\end{pmatrix} \right]$

Show that

(i) $$P(X_1>0,X_2>0,X_3>0)=\frac{1}{8}+\frac{\sin^{-1}\rho_{12}+\sin^{-1}\rho_{13}+\sin^{-1}\rho_{23}}{4\pi}$$

(ii) $$1+2\rho_{12}\rho_{13}\rho_{23}\ge \rho_{12}^2+\rho_{13}^2+\rho_{23}^2$$

My problem: I was solving test papers when I found this..

I had this sum done for a Bivariate Normal Distribution.

(X1, X2)~ BN( 0, 0,sigma_1^2, sigma_2^2, rho )

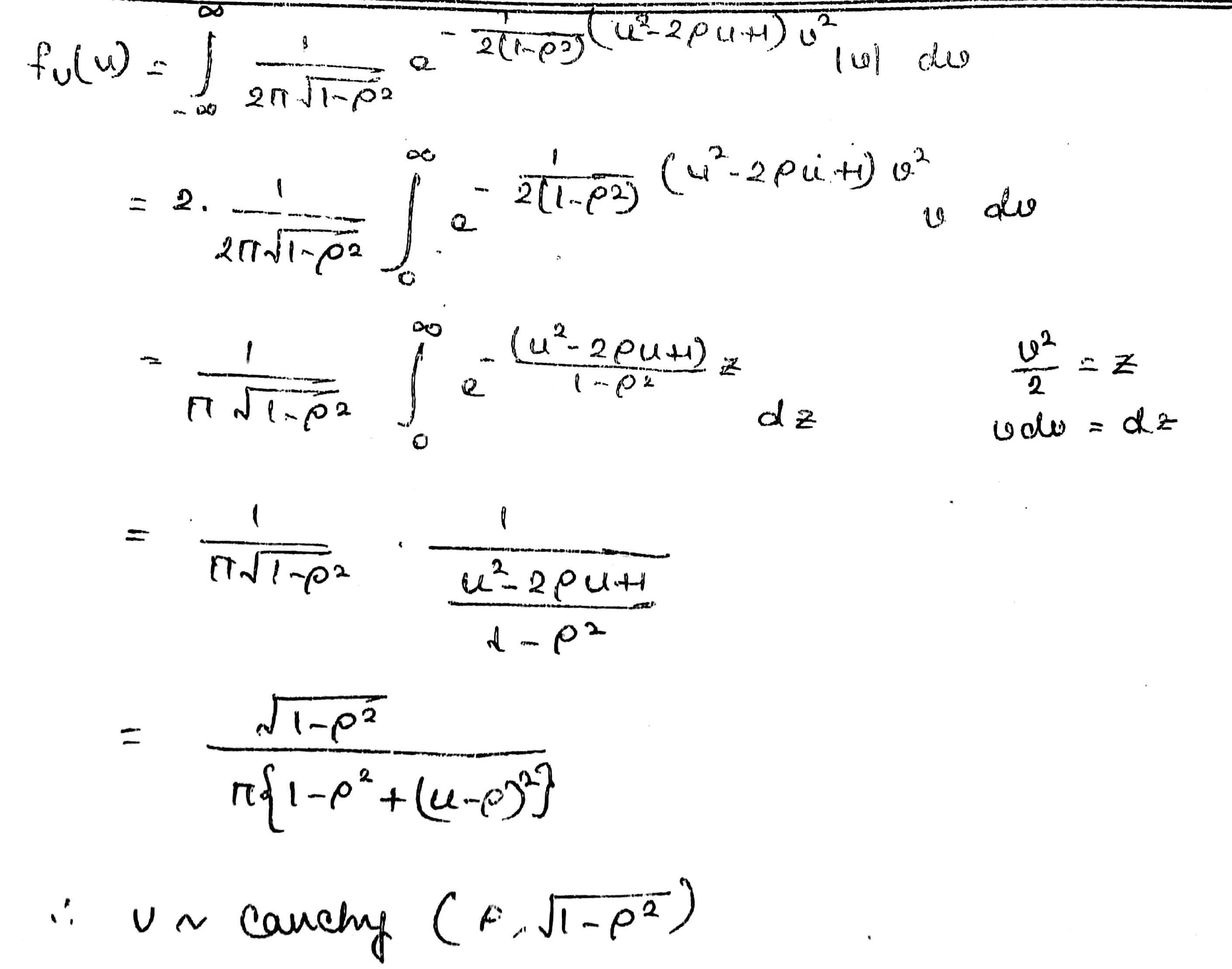
I know the formula of a Trivariate Normal Distribution.

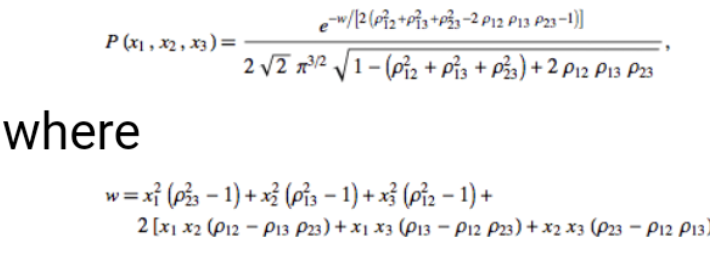
How can I prove the given relation (i) in the question, in a method like the Bivariate one was solved ?

And how do I solve part (ii) ? Please help. Thank you.

Answer 1

For the first question, indeed there is no need to work directly with the pdf of $(X_1,X_2,X_3)$.

Let the desired probability be $$p=P(X_1>0,X_2>0,X_3>0)$$

Analogous to the two-variable case, we have $$(X_1,X_2,X_3)\stackrel{d}{=}(-X_1,-X_2,-X_3)$$

So due to symmetry we must have

$$p=P(-X_1>0,-X_2>0,-X_3>0)=P(X_1<0,X_2<0,X_3<0)\tag{1}$$

Continuing from $(1)$,

\begin{align} 1-p&=P\left[\{X_1>0\}\cup\{X_2>0\}\cup\{X_3>0\}\right] \\\\&=P(X_1>0)+P(X_2>0)+P(X_3>0)-P(X_1>0,X_2>0) \\\\&\quad-P(X_2>0,X_3>0)-P(X_1>0,X_3>0)+p \\\\&=\frac{3}{2}-\left[\frac{3}{4}+\frac{1}{2\pi}(\sin^{-1}\rho_{12}+\sin^{-1}\rho_{23}+\sin^{-1}\rho_{13})\right]+p \end{align}

Or,

$$\\1-\left[\frac{3}{4}-\frac{1}{2\pi}(\sin^{-1}\rho_{12}+\sin^{-1}\rho_{23}+\sin^{-1}\rho_{13})\right]=2p$$

Finally,

$$p=\frac{1}{8}+\frac{1}{4\pi}(\sin^{-1}\rho_{12}+\sin^{-1}\rho_{23}+\sin^{-1}\rho_{13})$$

---

As a side note, I think that for the derivation of the expression for $P(X_1>0,X_2>0)$, it suffices to evaluate the corresponding double integral using a polar transformation. The details are not very messy. What you have done is perfectly fine by the way.