2
$\begingroup$

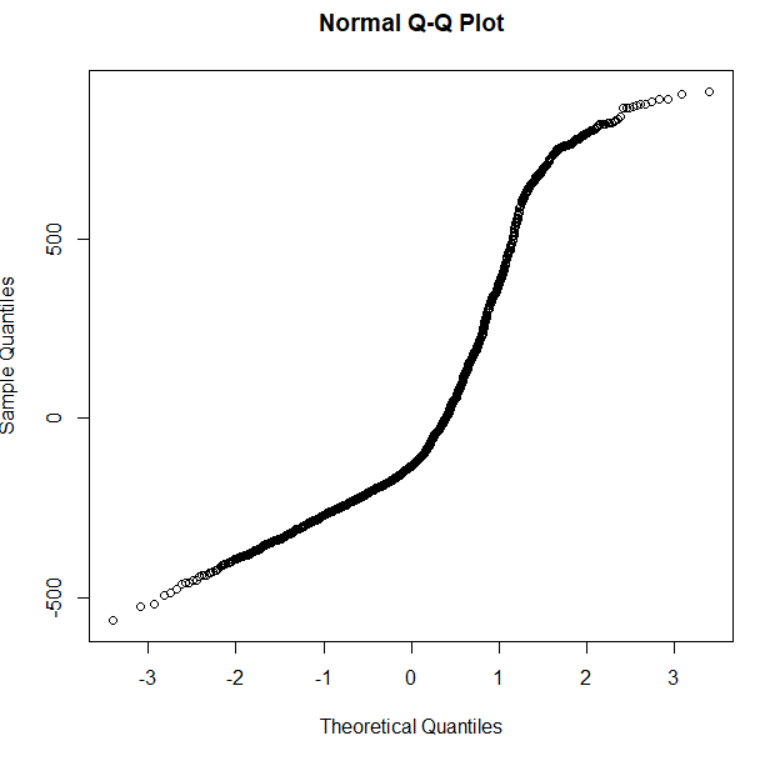
I have a repeated measures dataset with which I'm testing if individuals are consistent in their boldness scores (continuous variable) over time (trials). Towards this, I generated linear mixed effects model with boldness scores as the dependent variable, trials as fixed effects and individual IDs as random effects. However, when I checked the residuals of the fitted model, the assumption of normality is not met. I have attached the qqplot of the residuals.

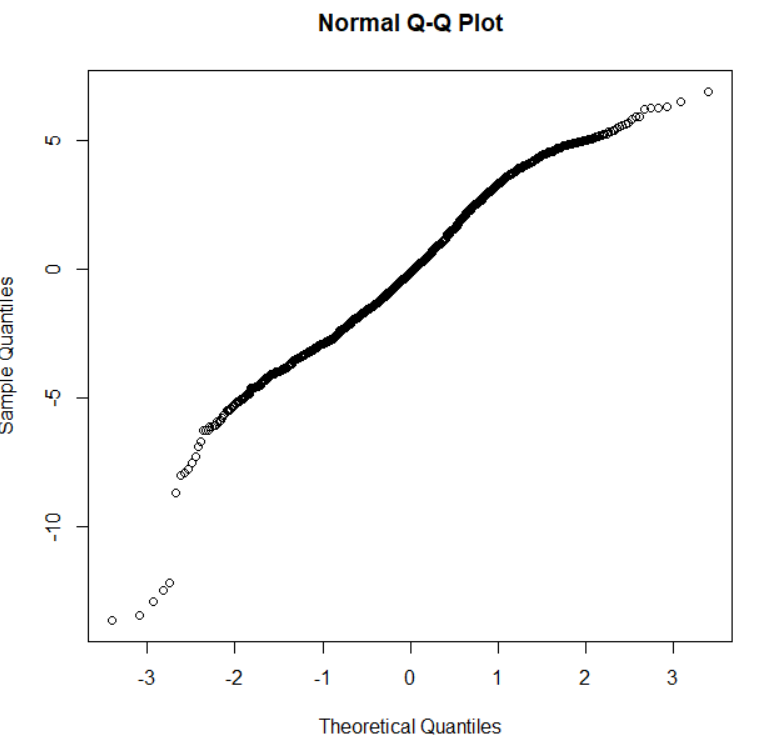
Before transformation enter image description here

I then transformed the boldness scores by boxcox method and again ran the model. The residuals seem to appear more normal now when compared to the residuals from the model with the non- transformed variable. However, even after transformation, shapiro tests show that the residuals are not normally distributed.

After transformation enter image description here

The skeweness of the residuals has also reduced from +1.111 to -0.24 after transformation.

Can I proceed with the model ignoring the assumption of normality of residuals? (see blogpost here which suggests linear mixed models are robust even if this assumption is not met).

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

The untransformed residuals appear to be bimodal. If so, this may indicate that there are two clusters within the dataset, and a transformation is very unlikely to result in a well-fitting model, and if it did there is a high chance that it would be over-fitted.

It is evident that the Box-Cox-transformed model residuals are not plausibly normal, as we would expect if the data are bimodal/clustered.

I would advise caution before proceeding. Typically this situation arises because the model is mis-specified. Some possible reasons include:

  • there could be one or more missing predictor variables that "explain" the bimodal structure (that is, they explain some separation in the data that the bimodality).
  • there could be one or more missing higher order terms of existing explanatory variables that "explain" the bimodal structure.
  • the underlying data generation model may not be approximated by a linear model in the range of the data observed

Possible solutions are:

  • Add other explanatory variables, if available, ideally informed by theory of the data generation process

  • Again informed by the underlying theory, try adding higher order terms.

  • Consider performing a cluster analysis or latent class analysis to identify clusters and introduce a new dummy variable which identifies which cluster an observation belongs to.

  • Consider a non-linear model, again informed by the underlying theory

  • Consider splitting the data so that one cluster is in one dataset and the other is in the other.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks much for the answer @Robert Long. The distribution of the response variable itself is bimodal, because all scores >20 min was coded as 1200 sec. So I removed all the 1200s, boxcox transformed the variable and obtained residuals after linear mixed model. The histogram of the residuals don't seem to be bimodal, and skewness has drastically reduced to -0.0009.Since the skew is so little, is the model acceptable for consideration? Unfortunately, I do not have additional explanatory variables. I also need mermod objects to fit random slope plots by sjplots package. Much appreciate any help. $\endgroup$
    – BP86
    Commented Dec 8, 2018 at 15:08

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.