In the study of causal inference on networks, what implications does assuming unconfoundedness have on homophily?

Question

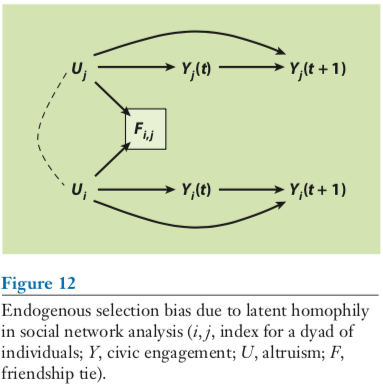
I read in a book on causal inference on networks that:

The unconfoundedness assumption does not rule out the presence of homophily, that is tendency of individuals who share similar characteristics to form ties. Homophily does not violate the unconfoudedness assumption in the cases where characteristics driving the homophily mechanism i) are included in the covariate set , ii) even if unobserved they do not affect the outcomes iii) they correspond to treament, that is, people who share the same treatment/exposure variable tend to form ties. The only situation where homophily is a threat to identification is when variables underlying the network formation process are not included in the covarite set and affect the outcome.

I am wondering if someone can give me more explanation on why we might even have reason to believe unconfoundedness can violate homophily. They seem to be two different things. What is it about the unconfoundedness assumption:

$$ Y(1),Y(0) \perp Z \mid X $$

that might even be related to homophily?

Answer 1

This matter is described by Elwert & Winship (2014, p45). I'll try to reproduce their argument but you should probably just read their paper, which is extremely accessible and a must-read for anyone thinking about causal inference.

Consider the following causal graph:

The important issue is that conditioning on $F$, the friendship tie, induces a non-causal association between $U_i$ and $U_j$, the causes of the ties (i.e., the "characteristics driving the homophily mechanism") because $F$ is a collider, and conditioning on a collider induces an association between its causes. Because these causes also cause the downstream outcomes, those outcomes become (noncausally) associated with each other, even when there is no causal relationship between those outcomes.

The statement you quoted claims three scenarios will not yield a violation of unconfoundedness. If you condition on $U$ (i.e., they are included in the covariate set), the noncausal associations between the outcomes will be blocked. If the $U$ do not cause the outcomes (i.e., the arrows from the $U$s to the $Y$s are removed), then no association is induced between the outcomes due to conditioning on $F$. If $U$ is the treatment, it is essentially being conditioned on, which again blocks the noncausal association between the outcomes. When there are no noncausal associations between the outcomes, we say there is unconfoundedness.

---

Elwert, F., & Winship, C. (2014). Endogenous Selection Bias: The Problem of Conditioning on a Collider Variable. Annual Review of Sociology, 40(1), 31–53. https://doi.org/10.1146/annurev-soc-071913-043455