2
$\begingroup$

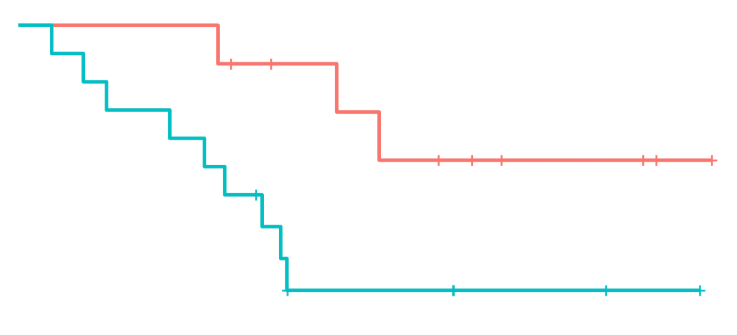
I have a simple question. I wanted to compare two independent survival curves, for example

enter image description here

Online resources tell me that the Mann-Whitney test can be used to test two independent groups or ordinal data.

My question is do I feed the test (1) the actual survival curves as seen on the picture, or (2) do I random sample the survival PMF and feed the random samples to the test?

My guess would be number (2) but the word ordinal confuses me.

Edit

There is no censoring - the image is just an example of two curves. Sorry for the confusion. Also - the curves are predicted so I don’t have exclusive events - that is the reason why I want to sample from the pmfs.

If sampling is the correct approach - do I have to order the event times before I input them in the test?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

As your plots show cases that have been censored (lost to follow-up before an event, indicated by the small cross marks along flat portions of the plots), the correct answer is:

(3) log-rank test or other survival analysis that takes the censoring into account.

As mentioned on the first page linked above, if there had been no censoring the log-rank test would provide the same results as a Mann-Whitney-Wilcoxon test. In that case you would simply provide the list of event times or ranks annotated as to treatment group. In your case you need to take censoring into account.

Improve this answer
$\endgroup$
3
  • $\begingroup$ The image is just an example - there is no censoring - that was unintended. Also I don’t have event times because I have predicted survival curves - that’s why I wanted to sample from each respective pmf. $\endgroup$
    – Edv Beq
    Commented Apr 26, 2019 at 13:06
  • $\begingroup$ @EdvBeq in that case please edit your question to say specifically what it is that you are trying to test. Testing for differences between predicted survival curves is different from testing empirical curves of the type you displayed. Testing differences between parametric continuous curves fit to data can be different from testing differences between Cox model semi-parametric fits based on different assumed covariate values. In none of those cases would Mann-Whitney likely be the test of choice. Without more details on what you are testing it will be hard to provide an answer. $\endgroup$
    – EdM
    Commented Apr 26, 2019 at 13:15
  • $\begingroup$ I added an edit to my post. I apologize for the confusion. I don’t have more info that what I added. I just want to test two hypothetical curves per say. $\endgroup$
    – Edv Beq
    Commented Apr 26, 2019 at 13:20

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.