1
$\begingroup$

[This question is mainly a reference request.]

I'm searching for a somehow concise and complete table of prototypical distributions that would allow a test person to easily choose which typical distribution he or she assumes or prefers

  • in a specific context, e.g. concerning income, health and life expectancy, well-being, political orientation (opinion), etc.

  • in his/her own country or world-wide

These are some examples I have found, but they are neither concise, complete nor well-displayed enough:

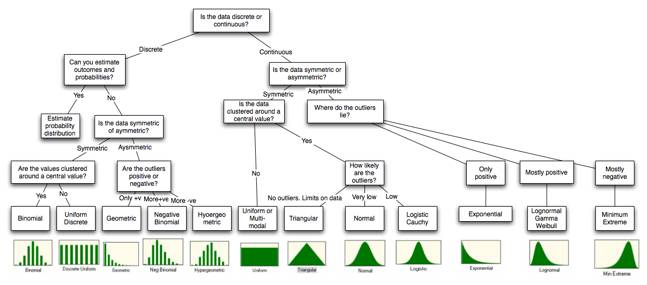enter image description here https://blog.cloudera.com/blog/2015/12/common-probability-distributions-the-data-scientists-crib-sheet/

enter image description here https://magesblog.com/post/2011-12-01-fitting-distributions-with-r/

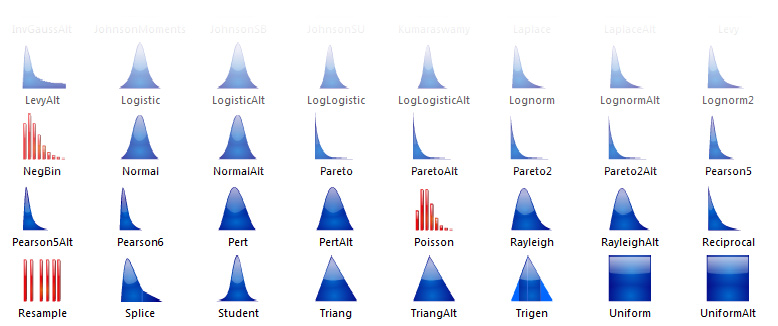enter image description here https://www.palisade.com/risk/monte_carlo_simulation.asp

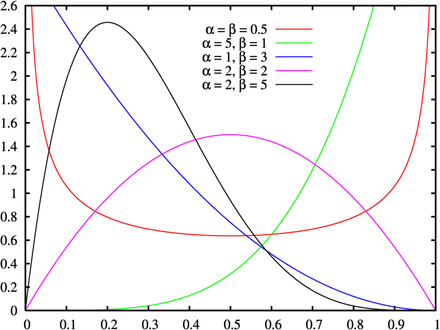enter image description here https://www.wikiwand.com/en/List_of_probability_distributions#/Continuous_distributions

Can anyone give me a link to a concise tabular overview of prototypical distributions (with one, two, three maxima and minima, preferrably with mean and median highlighted)?

If there is no such tabular overview around, I'll create it on my own.

To be more specific: In the context of my question there's no significant difference between Normal, Student's t and Weibull distribution. And none between Log Normal, Chi-Squared and Gamma. But between Gamma and Beta. And between Log Normal and Exponential (see first image above).

Improve this question
$\endgroup$
6
  • 2
    $\begingroup$ This would be a domain specific list, I'm afraid. There's infinite number of different functions that would pass as a probability density. Even the list of named distributions would very long, and most of its entries would be irrelevant to a reader. You need some way of filtering the list. It is best done in the domain context. You'll have to see what people are using in your field. $\endgroup$
    – Aksakal
    Commented May 16, 2019 at 17:34
  • $\begingroup$ My field is the domain of generic "fair", "just", "useful", "desirable", "preferable" distributions of any kind of "belongings" and "ways of being" - and these don't have to be uniform or normal. (Excuse me, I cannot be more specific than that.) $\endgroup$
    – Hans-Peter Stricker
    Commented May 16, 2019 at 17:44
  • $\begingroup$ Do you mean typical or prototypical? $\endgroup$
    – AdamO
    Commented May 16, 2019 at 17:47
  • $\begingroup$ @AdamO: Good question - I've thought about this and came to the conclusion that there is no deep difference. Which difference do you see? $\endgroup$
    – Hans-Peter Stricker
    Commented May 16, 2019 at 17:49
  • 1
    $\begingroup$ @Hans-PeterStricker it confuses me. Prototypical would mean something prespecified, a priori, or the result of a "thought experiment". Typical would mean something empirically discerned as a distinct type of object that commonly occured. $\endgroup$
    – AdamO
    Commented May 16, 2019 at 18:02

1 Answer 1

Reset to default
1
$\begingroup$

A rather comprehensive guide I just found is the Field Guide to Continuous Probability Distributions by Gavin E. Crooks.

At 210 pages it seems to contain a wealth of information, but as said in comments, for users a subject-specific guide might be more useful, and this is not.

Convincingly, this document comes with a version number and a long revision history, so it might have a future!

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.