To know the k value in Neyman Pearson lemma, do we need to know the alternate hypothesis. To what I understood (from articles like PenStateNotes), we could get value of k using null hypothesis and the required significance level alpha.
I am asking this because in one of our internal academic document, it was specified that we need to know even the alternate hypothesis to get the value of k.
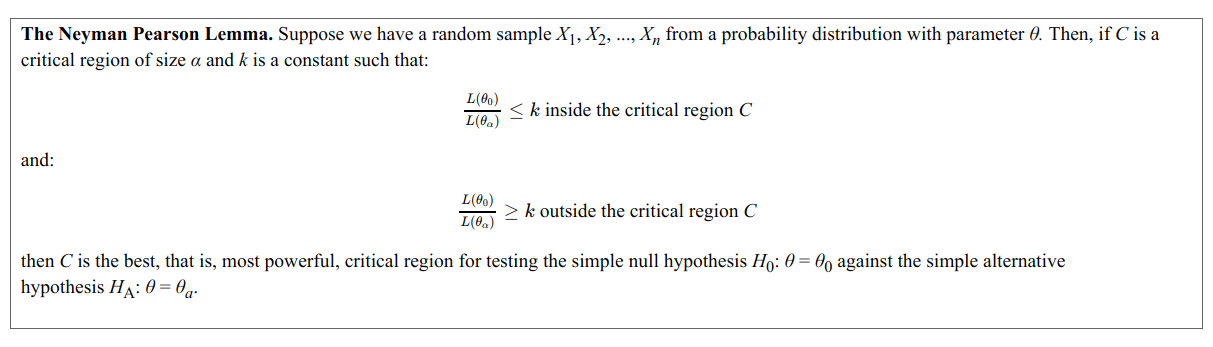
In a certain sense yes, you do "need to know" the alternative hypothesis, because in the Neyman-Person lemma it is required to compute the test statistic.
Specifically, let $X_1,\ldots,X_n$ be a random sample from the (continuous) probability distribution $p_\theta(x)$, $\theta$ unknown. The likelihood function is $L(\theta)=\prod_{i=1}^n p_\theta(x_i)$, where the $x_i$ are the observed sample values.
Suppose that you want to test the null hypothesis $H_0: \theta=\theta_0$ against the alternative $H_1: \theta=\theta_1$ for some $\theta_0\neq \theta_1$. Then the Neyman-Pearson lemma tells you to use the test statistic $T=L(\theta_0)/L(\theta_1)$. Note that to compute this, you need to know the value $\theta_1$ that defines the alternative $H_1$. Without knowing this, you could not compute $T$, and so not even $k$.
