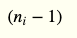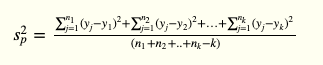2
$\begingroup$

Consider the pooled variance as described in this wiki article https://en.wikipedia.org/wiki/Pooled_variance :

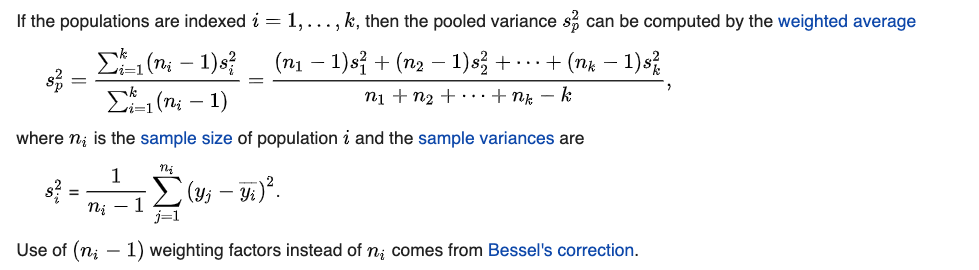
enter image description here

My observation is that the weighting terms

enter image description here

simply cancel out the Bessel's correction weighting factors. So then the result is simply

enter image description here

But then we no longer have count-based weightings on each of the variance terms! I must be missing something here - any insights/corrections?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

You are correct that this cancellation occurs, so for each sub-sample you get

$$(n_k-1) s_k^2 = \sum_{i=1}^{n_k} (y_{k,i} - \bar{y}_k)^2.$$

Each of these terms is a sum of $n_k$ squared deviations of the individual data points from the sub-sample mean. There is no need for any further weighting on the terms, since the summation already gives a quantity that is proportionate to the count of terms.

Improve this answer
$\endgroup$
1
  • $\begingroup$ oh ya - the summation is over the individual sample sizes! wrote it down but still "missed" it. thx. $\endgroup$
    – WestCoastProjects
    Commented Oct 3, 2019 at 13:32

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.