0
$\begingroup$

I do have three variables (Var1, Var2, and Var3) for which I have conducted a two-tailed pearson correlation (see also picture below).

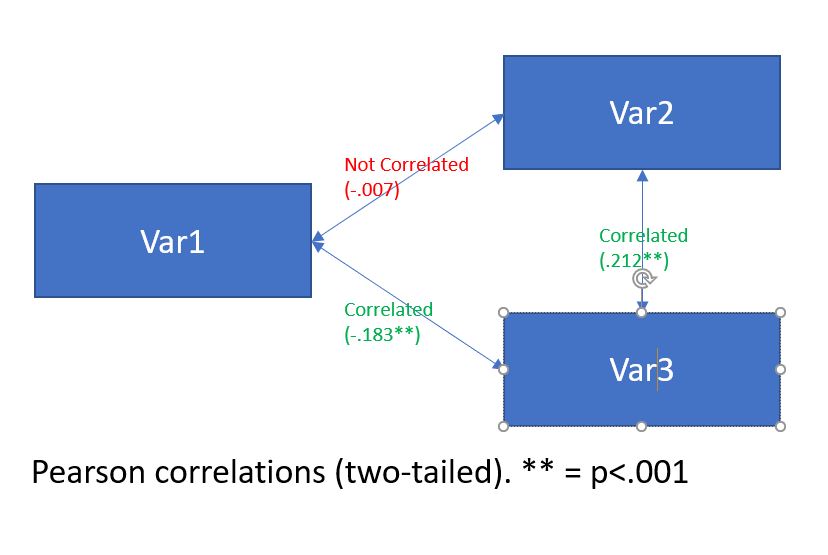
enter image description here

I am looking for a good explanation as to why:

  • Var 1 is not correlated with Var 2
  • Even though, Var 1 is correlated with Var3
  • Under the light that Var2 is correlated with Var3

I would have assumed that Var1 should also be correlated with Var2, as Var2 is correlated with Var3...

Kind Regards and thanks for the help

Improve this question
$\endgroup$
3
  • $\begingroup$ Suppose you started with $X_1$ and $X_2$ and $\epsilon$ all independent and then set $X_3=X_2-X_1+\epsilon$. Then you might get something like what you have seen with correlations between $X_3$ and the other two, but not between $X_1$ and $X_2$ $\endgroup$
    – Henry
    Commented Feb 10, 2020 at 8:17
  • $\begingroup$ Thanks for the answer Henry: You need to help me out a little bit. Unfortunately, I am not a mathematician. Is there a simple explanation for the behaviour which I described above? Thx $\endgroup$
    – Raphael
    Commented Feb 10, 2020 at 8:28
  • 1
    $\begingroup$ A scatter plot matrix with all pairwise scatter plots should also help, $\endgroup$
    – Nick Cox
    Commented Feb 10, 2020 at 10:00

1 Answer 1

Reset to default
2
$\begingroup$

A correlation expresses an association between two variables. One can find the variance that's shared between two variables by squaring the correlation coefficient (e.g., shared variance = $r^2$.) So, the shared variance between variable 1 and 3 in your diagram is 0.033, and the shared variance between variable 3 and 2 is 0.045. That means, there's 1-0.033 (.967) of the variance in variables 1 and 3 left unexplained by their relationship, and 1-0.045 (0.955) of the variance in variables 2 and 3 left unexplained by their relationship. Given the tiny components of explained variance, it's very possible that there is no overlap between variables 1 and 2, they're independent.

Let me give you a real-world example. Let's say people who love iPhones also love Wired Magazine. And, people who love Android phones love Wired magazine. However, people who love iPhones don't necessarily love Android phones (some do, some don't.) There doesn't have to be a relationship between two variables for them to both prove helpful in predicting another variable. They can each explain part of the variance.

Basil also presents an excellent plain-english explanation in this thread: If A and B are correlated with C, why are A and B not necessarily correlated?

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.