I want to use ANCOVA to test whether means on a dependent variable (DV) differ between 3 groups (the independent variable, IV) while adding 3 variables as covariates (CV).
One assumption of ANCOVA is that the regression slopes of a covariate should be homogeneous across levels of the IV.
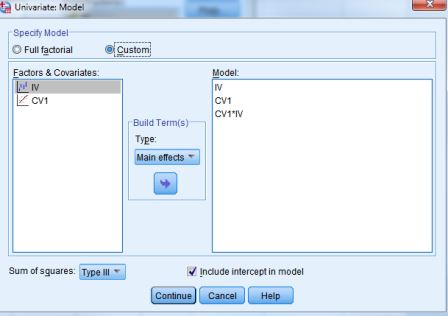
I learned that when having one covariate you could test this assumption, by running the model with the main effects of the IV and CV while also adding the interaction effect best of the IV and CV to the model an check, whether the interaction is indeed not significant.
Here an example in SPSS:
1. How should this assumption be tested with multiple covariates? (2. and why?)
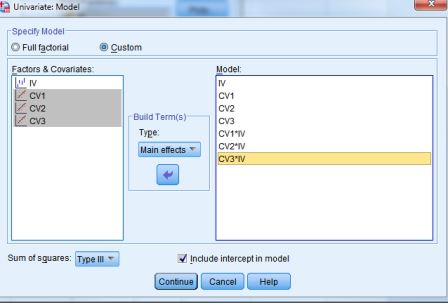
Could you do it by running one model including the IV, all CVs and all IV*CV interaction terms?
SPSS example:
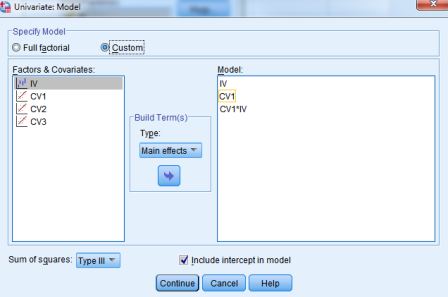
Or should this assumption better be tested separately for each covariate? Thus testing the model with main effects for the IV and CV1 and interaction of IV and CV1 repeat this with CV2 and CV3?
Thus repeating the following with CV2 and CV3:
I assumed that you should test it using a model with all CVs and CV*IV interaction effects since you already assume that all CVs are related to your DV and therefore need to be accounted for when testing whether the effect of a particular CV on the DV is equal across levels of the IV. Unfortunately all sources I looked up until now discuss testing this assumption in contexts where only one covariate is used.