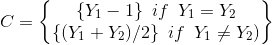Question about calculating confidence intervals

Question

I am reading about confidence intervals and got stuck with this example from L. Wasserman's book titled "All of Statistics". Could anybody explain why P_Q(θ ∈ C) = 3/4 in this example? Below is the paragraph from the book:

Let θ be a fixed, known real number and let X₁, X₂ be independent random variables such that P(X_i = 1) = P(X_i = -1) = 1/2. Now define Y_i = θ + X_i and suppose that you only observe Y₁ and Y₂. Define the following interval that actually contains only one point:

You can check that, no matter what θ is, we have P_θ(θ ∈ C) = 3/4 so this is a 75 percent confidence interval. Suppose we now do the experiment and we get Y₁ = 15 and Y₂ = 17. Then our 75 percent confidence interval is {16}. However, we are certain that θ = 16. If you wanted to make a probability statement about θ you would probably say that P(θ ∈ C|Y₁, Y₂) = 1. There is nothing wrong with saying that {16} is a 75 percent confidence interval. But is it not a probability statement about θ.

Answer 1

You can dissociate cases :

• if $X_1 \neq X_2$, which happens with probability $\frac{1}{2}$, then $X_1 = -X_2$ (since $X$ can only be $1$ or $-1$) and $Y_1 \neq Y_2$. So $C = \{\frac{Y_1 + Y_2}{2}\} = \{\theta\}$. So $\theta \in C$.

• if $X_1 = X_2 = 1$, which happens with probability $\frac{1}{4}$, then $Y_1 = Y_2 = \theta + 1$ and $C = \{\theta\}$. So $\theta \in C$.

• if $X_1 = X_2 = -1$, which happens with probability $\frac{1}{4}$, then $Y_1 = Y_2 = \theta - 1$, and $C = \{\theta - 2\}$. So $\theta \notin C$.

So the only possible case in which $\theta \notin C$ is when $X_1 = X_2 = -1$, which happens with probability $\frac{1}{4}$, so $P(\theta \in C) = 1 - \frac{1}{4} = \frac{3}{4}$

I think the point of Wasserman here is that the randomness lies in $C$ and not $\theta$. And indeed in the different cases considered, each time it is the confidence intervall $C$ which changes, not $\theta$.