Instrumental variables- unconfoundness vs exclusion restriction

Question

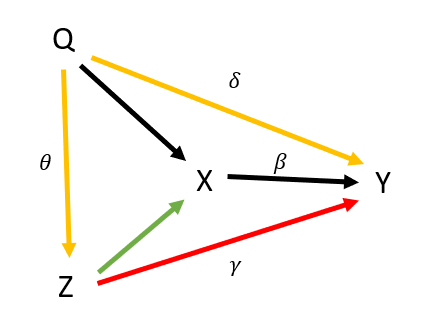
I typically see people refer to exclusion restriction as the underlying assumption needed for causal interpretation of IV. But are there actually two assumptions needed?

say we have $y_{i,t} = \beta x_{i,t} + \epsilon_{i,t}$

and $z_{i,t}$ is the instrument. Don't we need:

1) The instrument has to be un-correlated with any other unobserved determinant of $Y,$ and 2) It has to affect $Y$ only by changing $X.$

Isn't only the latter the exclusion restriction? And without that, if the first is satisfied, the reduced form/intent to treat (ITT) should still be causal, correct? The second just allows you to interpret it as the effect of $X?$

Does $\operatorname{Cov}(z,\epsilon)=0$ incorporate both above?

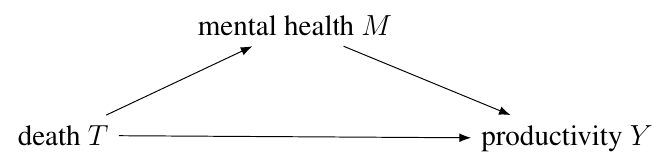
With an example I have seen, people use the surprise death of a highly productive worker to calculate the effect of losing a highly productive collaborator on others' productivity. In this case, if the death was a surprise, surely 1 is satisfied. But, if the death causes mental health problems for the collaborators which then affect their productivity, would this then mean the exclusion restriction failed, but the instrument is still un-confounded, and therefore the reduced from/ITT is still causal?

Answer 1

You are right. Adding an explicit, potentially confounding variable $Q$ to our analysis may help illustrate why both assumptions are necessary for $Cov(\epsilon, Z) = 0$

$$ Y_i = \beta X_i + \gamma Z_i + \delta Q_i + u_i $$ $$ Z_i = \theta Q_i + v_i $$

If we let the instrument and the potential confounder be part of the error term in the outcome equation, we have

$$ Y_i = \beta X_i + \epsilon_i $$ $$ \epsilon_i = \gamma Z_i + \delta Q_i + u_i $$

Substituting for $Z$ and $\epsilon$ in the covariance formula and assuming $Q$, $u$, and $v$ are uncorrelated with each other

$$ Cov(Z_i, \epsilon_i) = \left(\gamma\theta^2 + \delta\theta\right)Var(Q_i) + \gamma Var(v_i) $$

We see that unless $ \left(\gamma\theta^2 + \delta\theta\right)Var(Q_i) $ happens to equal $ -\gamma Var(v_i) $ we requrire both $\gamma = 0$ (the instrument satisfies the exclusion restriction) and $\theta = 0 $ or $\delta = 0 $ (the isntrument is exogenous, aka as good as randomly assigned) for $Cov(\epsilon, Z) = 0$. To avoid confusion, I think it’s better to refer to the assumption that $Cov(\epsilon, Z) = 0$ as the validity of the instrument. My impression is also that it is fairly commmon jargon to say that the instrument has to be both relevant and valid.

A causal diagram is good for explaining the difference between exogeneity an the exlusion restriction and why both are necessary.

Here, there is a single confounder for the effect of the treatment (X) on the outcome (Y). The instrument (Z) is not exogenous if it is also affected by the confounder. The instrument does not satisfy the exlusion restriction if it has a direct effect on the outcome.

Answer 2

On page 86 of Causal Inference in Statistics: A Primer, by Pearl, Glymour, and Jewell, the authors state that

A variable is called an "instrument" if it is $d$-separated from $Y$ in $G_\alpha$ and, it is $d$-connected to $X.$

Note here that $G_\alpha$ is the graph obtained from the initial causal graph by removing the arrow from $X$ to $Y.$ These conditions are different from your #1 assumption, as correlation might be there or it might not. The quoted condition is equivalent to your #2. Without this condition, the variable would not be an instrument, and the ensuing analysis would be invalid.

In the case of the death of the highly productive worker, surely that is a mediation situation, like this:

Nothing in sight could be used as an instrumental variable, though you definitely could use mediation analysis.

Answer 3

You're mixing up some terminology. "Exclusion restriction" means your Condition 1)---all other variables in the economy can be "excluded" from your model. Put differently, zero restriction can be imposed on their coefficients.

The requirements of IV are

1. Exclusion Restriction / Exogeneity: $Cov(z, \epsilon) = 0$.

2. Relevance: $Cov(z,x) \neq 0$.

Conditions 1 and 2 together imply, empirically, that "$z$ only affects $y$ through $x$". Often the relevance condition is empirically clear and the focus is on the exogeneity condition---as in your example.

For your example, you are raising an objection to the exogeneity of the proposed instrument. You are suggesting certain component of $\epsilon$ (co-worker productivity) that could be correlated with the instrument---i.e. the exclusion restriction does not hold.

It is up to the empirical researcher who proposed the instrument to address such issues. If you, or anyone else, propose an IV, it is your responsibility to justify/defend exogeneity of your instrument to the professional community.

(In this case, one hypothetical defense is that the suggested impact on co-worker productivity is argued to not occur in practice.)